how to tell if a hypothesis is left tailed

How to Identify a Left Tailed Test vs. a Right Tailed Test

In statistics, we use hypothesis tests to determine whether some claim about a population parameter is true or not.

Whenever we perform a hypothesis test, we always write a null hypothesis and an alternative hypothesis , which take the following forms:

H 0 (Null Hypothesis): Population parameter = ≤, ≥ some value

H A (Alternative Hypothesis): Population parameter <, >, ≠ some value

There are three different types of hypothesis tests:

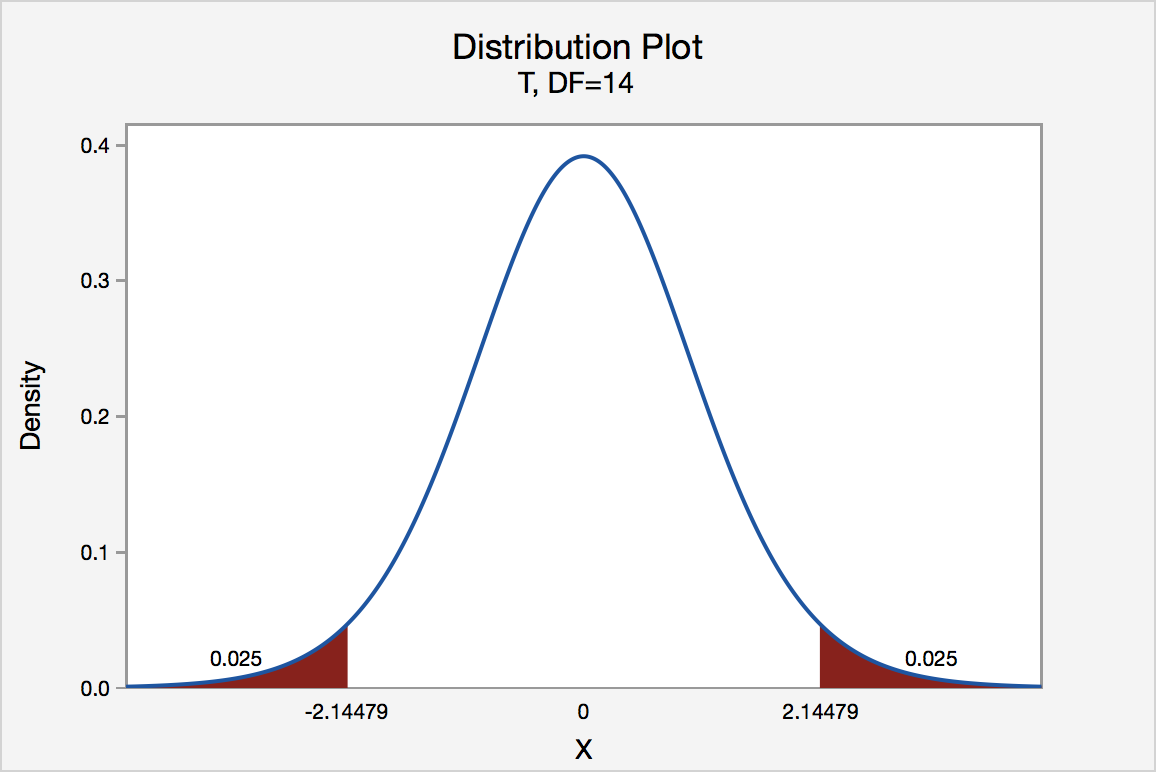
• Two-tailed test: The alternative hypothesis contains the “≠” sign
• Left-tailed test: The alternative hypothesis contains the “<” sign
• Right-tailed test: The alternative hypothesis contains the “>” sign

Notice that we only have to look at the sign in the alternative hypothesis to determine the type of hypothesis test.

Left-tailed test: The alternative hypothesis contains the “<” sign   Right-tailed test: The alternative hypothesis contains the “>” sign

The following examples show how to identify left-tailed and right-tailed tests in practice.

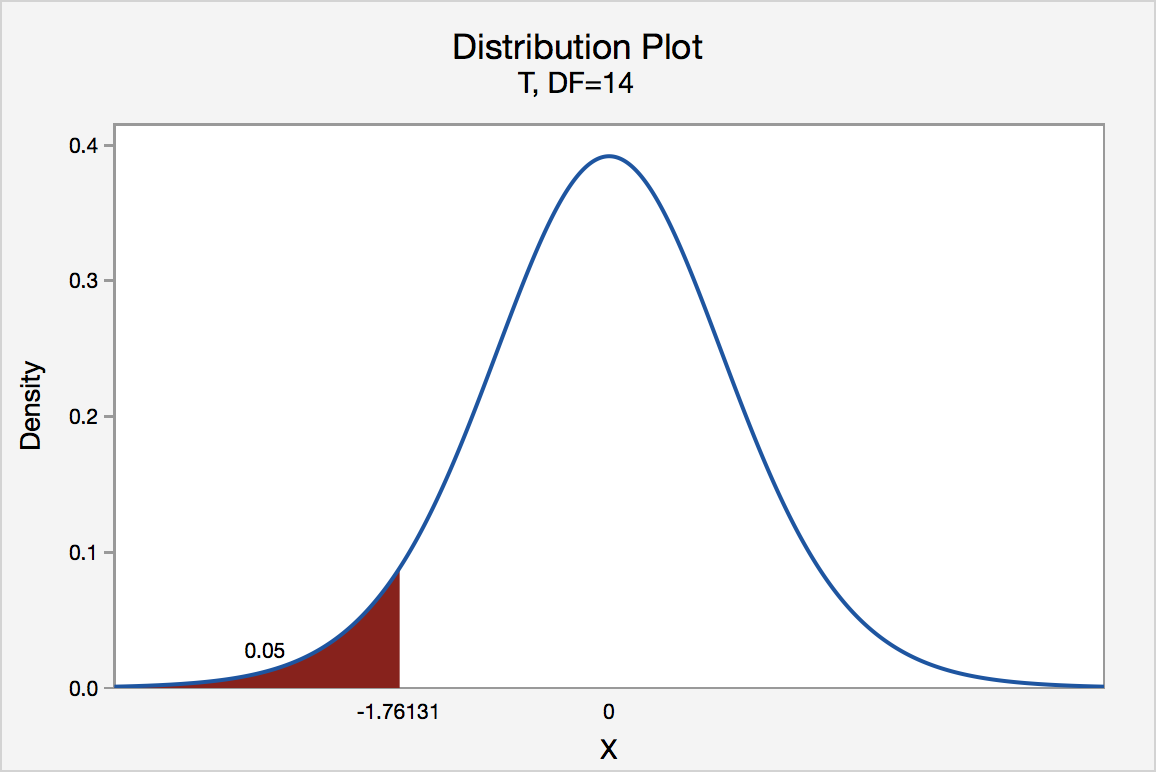
Example: Left-Tailed Test

Suppose it’s assumed that the average weight of a certain widget produced at a factory is 20 grams. However, one inspector believes the true average weight is less than 20 grams.

To test this, he weighs a simple random sample of 20 widgets and obtains the following information:

• n = 20 widgets
• x = 19.8 grams
• s = 3.1 grams

He then performs a hypothesis test using the following null and alternative hypotheses:

H 0 (Null Hypothesis): μ ≥ 20 grams

H A (Alternative Hypothesis): μ < 20 grams

The test statistic is calculated as:

• t  = ( x – µ) / (s/√ n )
• t = (19.8-20) / (3.1/√ 20 )

According to the t-Distribution table , the t critical value at α = .05 and n-1 = 19 degrees of freedom is – 1.729 .

Since the test statistic is not less than this value, the inspector fails to reject the null hypothesis. He does not have sufficient evidence to say that the true mean weight of widgets produced at this factory is less than 20 grams.

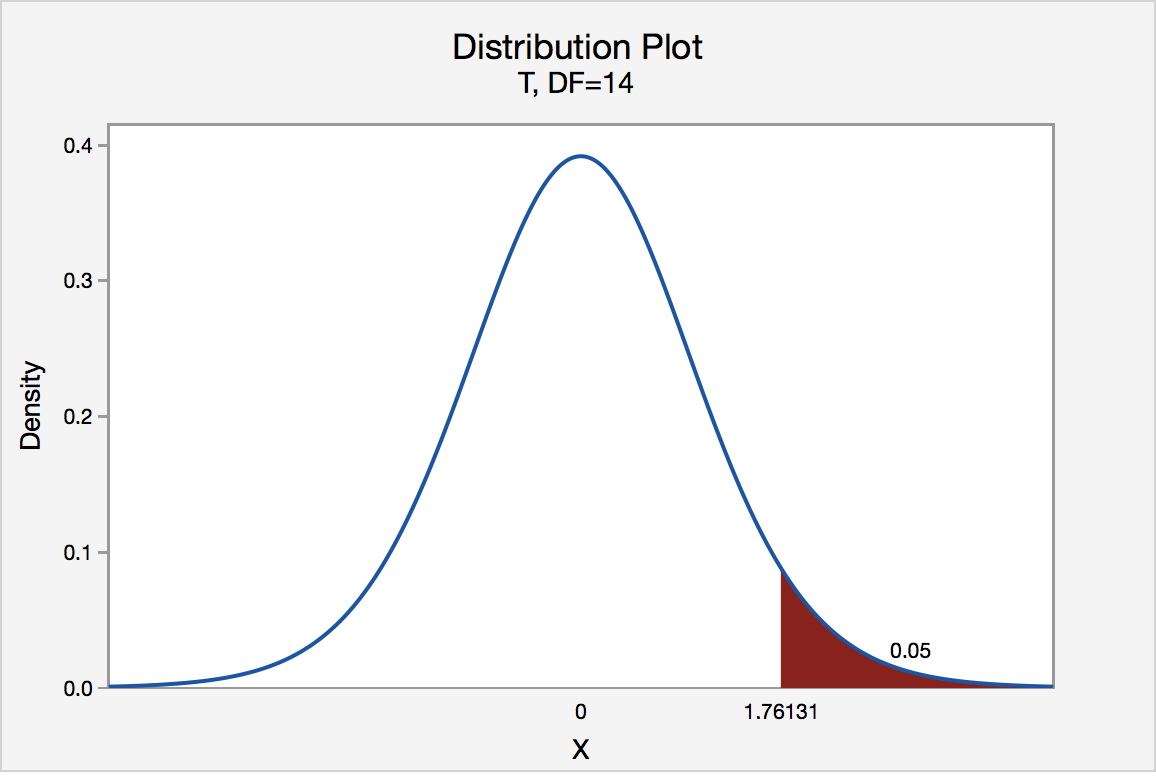
Example: Right-Tailed Test

Suppose it’s assumed that the average height of a certain species of plant is 10 inches tall. However, one botanist claims the true average height is greater than 10 inches.

To test this claim, she goes out and measures the height of a simple random sample of 15 plants and obtains the following information:

• n = 15 plants
• x = 11.4 inches
• s = 2.5 inches

She then performs a hypothesis test using the following null and alternative hypotheses:

H 0 (Null Hypothesis): μ ≤ 10 inches

H A (Alternative Hypothesis): μ > 10 inches

• t = (11.4-10) / (2.5/√ 15 )

According to the t-Distribution table , the t critical value at α = .05 and n-1 = 14 degrees of freedom is 1.761 .

Since the test statistic is greater than this value, the botanist can reject the null hypothesis. She has sufficient evidence to say that the true mean height for this species of plant is greater than 10 inches.

Additional Resources

How to Read the t-Distribution Table One Sample t-test Calculator Two Sample t-test Calculator

Featured Posts

Hey there. My name is Zach Bobbitt. I have a Masters of Science degree in Applied Statistics and I’ve worked on machine learning algorithms for professional businesses in both healthcare and retail. I’m passionate about statistics, machine learning, and data visualization and I created Statology to be a resource for both students and teachers alike.  My goal with this site is to help you learn statistics through using simple terms, plenty of real-world examples, and helpful illustrations.

3 Replies to “How to Identify a Left Tailed Test vs. a Right Tailed Test”

This was so helpful you are a life saver. Thank you so much

Left-tailed test example, -2.885 is less than -1.729, why you mention -2.885 is “not lesss” …

Isn’t it -0.2885 instead of -2.885?

Leave a Reply Cancel reply

Your email address will not be published. Required fields are marked *

Join the Statology Community

Sign up to receive Statology's exclusive study resource: 100 practice problems with step-by-step solutions. Plus, get our latest insights, tutorials, and data analysis tips straight to your inbox!

By subscribing you accept Statology's Privacy Policy.

How to Identify a Left Tailed Test vs. a Right Tailed Test

In statistics, we use hypothesis tests to determine whether some claim about a population parameter is true or not.

Whenever we perform a hypothesis test, we always write a null hypothesis and an alternative hypothesis , which take the following forms:

H 0 (Null Hypothesis): Population parameter = ≤, ≥ some value

H A (Alternative Hypothesis): Population parameter , ≠ some value

There are three different types of hypothesis tests:

• Two-tailed test: The alternative hypothesis contains the “≠” sign
• Left-tailed test: The alternative hypothesis contains the “
• Right-tailed test: The alternative hypothesis contains the “>” sign

Notice that we only have to look at the sign in the alternative hypothesis to determine the type of hypothesis test.

Left-tailed test: The alternative hypothesis contains the “   Right-tailed test: The alternative hypothesis contains the “>” sign

The following examples show how to identify left-tailed and right-tailed tests in practice.

Example: Left-Tailed Test

Suppose it’s assumed that the average weight of a certain widget produced at a factory is 20 grams. However, one inspector believes the true average weight is less than 20 grams.

To test this, he weighs a simple random sample of 20 widgets and obtains the following information:

• n = 20 widgets
• x = 19.8 grams
• s = 3.1 grams

He then performs a hypothesis test using the following null and alternative hypotheses:

H 0 (Null Hypothesis): μ ≥ 20 grams

H A (Alternative Hypothesis): μ

The test statistic is calculated as:

• t  = ( x – µ) / (s/√ n )
• t = (19.8-20) / (3.1/√ 20 )

According to the t-Distribution table , the t critical value at α = .05 and n-1 = 19 degrees of freedom is – 1.729 .

Since the test statistic is not less than this value, the inspector fails to reject the null hypothesis. He does not have sufficient evidence to say that the true mean weight of widgets produced at this factory is less than 20 grams.

Example: Right-Tailed Test

Suppose it’s assumed that the average height of a certain species of plant is 10 inches tall. However, one botanist claims the true average height is greater than 10 inches.

To test this claim, she goes out and measures the height of a simple random sample of 15 plants and obtains the following information:

• n = 15 plants
• x = 11.4 inches
• s = 2.5 inches

She then performs a hypothesis test using the following null and alternative hypotheses:

H 0 (Null Hypothesis): μ ≤ 10 inches

H A (Alternative Hypothesis): μ > 10 inches

• t = (11.4-10) / (2.5/√ 15 )

According to the t-Distribution table , the t critical value at α = .05 and n-1 = 14 degrees of freedom is 1.761 .

Since the test statistic is greater than this value, the botanist can reject the null hypothesis. She has sufficient evidence to say that the true mean height for this species of plant is greater than 10 inches.

Additional Resources

How to Read the t-Distribution Table One Sample t-test Calculator Two Sample t-test Calculator

Joint Frequency: Definition & Examples

G-test of goodness of fit: definition + example, related posts, how to normalize data between -1 and 1, vba: how to check if string contains another..., how to interpret f-values in a two-way anova, how to create a vector of ones in..., how to find the mode of a histogram..., how to find quartiles in even and odd..., how to determine if a probability distribution is..., what is a symmetric histogram (definition & examples), how to calculate sxy in statistics (with example), how to calculate sxx in statistics (with example).

• Skip to secondary menu
• Skip to main content
• Skip to primary sidebar

Statistics By Jim

Making statistics intuitive

One-Tailed and Two-Tailed Hypothesis Tests Explained

By Jim Frost 61 Comments

Choosing whether to perform a one-tailed or a two-tailed hypothesis test is one of the methodology decisions you might need to make for your statistical analysis. This choice can have critical implications for the types of effects it can detect, the statistical power of the test, and potential errors.

In this post, you’ll learn about the differences between one-tailed and two-tailed hypothesis tests and their advantages and disadvantages. I include examples of both types of statistical tests. In my next post, I cover the decision between one and two-tailed tests in more detail.

What Are Tails in a Hypothesis Test?

First, we need to cover some background material to understand the tails in a test. Typically, hypothesis tests take all of the sample data and convert it to a single value, which is known as a test statistic. You’re probably already familiar with some test statistics. For example, t-tests calculate t-values . F-tests, such as ANOVA, generate F-values . The chi-square test of independence and some distribution tests produce chi-square values. All of these values are test statistics. For more information, read my post about Test Statistics .

These test statistics follow a sampling distribution. Probability distribution plots display the probabilities of obtaining test statistic values when the null hypothesis is correct. On a probability distribution plot, the portion of the shaded area under the curve represents the probability that a value will fall within that range.

The graph below displays a sampling distribution for t-values. The two shaded regions cover the two-tails of the distribution.

Keep in mind that this t-distribution assumes that the null hypothesis is correct for the population. Consequently, the peak (most likely value) of the distribution occurs at t=0, which represents the null hypothesis in a t-test. Typically, the null hypothesis states that there is no effect. As t-values move further away from zero, it represents larger effect sizes. When the null hypothesis is true for the population, obtaining samples that exhibit a large apparent effect becomes less likely, which is why the probabilities taper off for t-values further from zero.

Related posts : How t-Tests Work and Understanding Probability Distributions

Critical Regions in a Hypothesis Test

In hypothesis tests, critical regions are ranges of the distributions where the values represent statistically significant results. Analysts define the size and location of the critical regions by specifying both the significance level (alpha) and whether the test is one-tailed or two-tailed.

Consider the following two facts:

• The significance level is the probability of rejecting a null hypothesis that is correct.
• The sampling distribution for a test statistic assumes that the null hypothesis is correct.

Consequently, to represent the critical regions on the distribution for a test statistic, you merely shade the appropriate percentage of the distribution. For the common significance level of 0.05, you shade 5% of the distribution.

Related posts : Significance Levels and P-values and T-Distribution Table of Critical Values

Two-Tailed Hypothesis Tests

Two-tailed hypothesis tests are also known as nondirectional and two-sided tests because you can test for effects in both directions. When you perform a two-tailed test, you split the significance level percentage between both tails of the distribution. In the example below, I use an alpha of 5% and the distribution has two shaded regions of 2.5% (2 * 2.5% = 5%).

When a test statistic falls in either critical region, your sample data are sufficiently incompatible with the null hypothesis that you can reject it for the population.

In a two-tailed test, the generic null and alternative hypotheses are the following:

• Null : The effect equals zero.
• Alternative :  The effect does not equal zero.

The specifics of the hypotheses depend on the type of test you perform because you might be assessing means, proportions, or rates.

Example of a two-tailed 1-sample t-test

Suppose we perform a two-sided 1-sample t-test where we compare the mean strength (4.1) of parts from a supplier to a target value (5). We use a two-tailed test because we care whether the mean is greater than or less than the target value.

To interpret the results, simply compare the p-value to your significance level. If the p-value is less than the significance level, you know that the test statistic fell into one of the critical regions, but which one? Just look at the estimated effect. In the output below, the t-value is negative, so we know that the test statistic fell in the critical region in the left tail of the distribution, indicating the mean is less than the target value. Now we know this difference is statistically significant.

We can conclude that the population mean for part strength is less than the target value. However, the test had the capacity to detect a positive difference as well. You can also assess the confidence interval. With a two-tailed hypothesis test, you’ll obtain a two-sided confidence interval. The confidence interval tells us that the population mean is likely to fall between 3.372 and 4.828. This range excludes the target value (5), which is another indicator of significance.

Advantages of two-tailed hypothesis tests

You can detect both positive and negative effects. Two-tailed tests are standard in scientific research where discovering any type of effect is usually of interest to researchers.

One-Tailed Hypothesis Tests

One-tailed hypothesis tests are also known as directional and one-sided tests because you can test for effects in only one direction. When you perform a one-tailed test, the entire significance level percentage goes into the extreme end of one tail of the distribution.

In the examples below, I use an alpha of 5%. Each distribution has one shaded region of 5%. When you perform a one-tailed test, you must determine whether the critical region is in the left tail or the right tail. The test can detect an effect only in the direction that has the critical region. It has absolutely no capacity to detect an effect in the other direction.

In a one-tailed test, you have two options for the null and alternative hypotheses, which corresponds to where you place the critical region.

You can choose either of the following sets of generic hypotheses:

• Null : The effect is less than or equal to zero.
• Alternative : The effect is greater than zero.

• Null : The effect is greater than or equal to zero.
• Alternative : The effect is less than zero.

Again, the specifics of the hypotheses depend on the type of test you perform.

Notice how for both possible null hypotheses the tests can’t distinguish between zero and an effect in a particular direction. For example, in the example directly above, the null combines “the effect is greater than or equal to zero” into a single category. That test can’t differentiate between zero and greater than zero.

Example of a one-tailed 1-sample t-test

Suppose we perform a one-tailed 1-sample t-test. We’ll use a similar scenario as before where we compare the mean strength of parts from a supplier (102) to a target value (100). Imagine that we are considering a new parts supplier. We will use them only if the mean strength of their parts is greater than our target value. There is no need for us to differentiate between whether their parts are equally strong or less strong than the target value—either way we’d just stick with our current supplier.

Consequently, we’ll choose the alternative hypothesis that states the mean difference is greater than zero (Population mean – Target value > 0). The null hypothesis states that the difference between the population mean and target value is less than or equal to zero.

To interpret the results, compare the p-value to your significance level. If the p-value is less than the significance level, you know that the test statistic fell into the critical region. For this study, the statistically significant result supports the notion that the population mean is greater than the target value of 100.

Confidence intervals for a one-tailed test are similarly one-sided. You’ll obtain either an upper bound or a lower bound. In this case, we get a lower bound, which indicates that the population mean is likely to be greater than or equal to 100.631. There is no upper limit to this range.

A lower-bound matches our goal of determining whether the new parts are stronger than our target value. The fact that the lower bound (100.631) is higher than the target value (100) indicates that these results are statistically significant.

This test is unable to detect a negative difference even when the sample mean represents a very negative effect.

Advantages and disadvantages of one-tailed hypothesis tests

One-tailed tests have more statistical power to detect an effect in one direction than a two-tailed test with the same design and significance level. One-tailed tests occur most frequently for studies where one of the following is true:

• Effects can exist in only one direction.
• Effects can exist in both directions but the researchers only care about an effect in one direction. There is no drawback to failing to detect an effect in the other direction. (Not recommended.)

The disadvantage of one-tailed tests is that they have no statistical power to detect an effect in the other direction.

As part of your pre-study planning process, determine whether you’ll use the one- or two-tailed version of a hypothesis test. To learn more about this planning process, read 5 Steps for Conducting Scientific Studies with Statistical Analyses .

This post explains the differences between one-tailed and two-tailed statistical hypothesis tests. How these forms of hypothesis tests function is clear and based on mathematics. However, there is some debate about when you can use one-tailed tests. My next post explores this decision in much more depth and explains the different schools of thought and my opinion on the matter— When Can I Use One-Tailed Hypothesis Tests .

If you’re learning about hypothesis testing and like the approach I use in my blog, check out my Hypothesis Testing book! You can find it at Amazon and other retailers.

Share this:

Reader Interactions

August 23, 2024 at 1:28 pm

Thank so much. This is very helpfull

June 26, 2022 at 12:14 pm

Hi, Can help me with figuring out the null and alternative hypothesis of the following statement? Some claimed that the real average expenditure on beverage by general people is at least $10.

February 19, 2022 at 6:02 am

thank you for the thoroughly explanation, I’m still strugling to wrap my mind around the t-table and the relation between the alpha values for one or two tail probability and the confidence levels on the bottom (I’m understanding it so wrongly that for me it should be the oposite, like one tail 0,05 should correspond 95% CI and two tailed 0,025 should correspond to 95% because then you got the 2,5% on each side). In my mind if I picture the one tail diagram with an alpha of 0,05 I see the rest 95% inside the diagram, but for a one tail I only see 90% CI paired with a 5% alpha… where did the other 5% go? I tried to understand when you said we should just double the alpha for a one tail probability in order to find the CI but I still cant picture it. I have been trying to understand this. Like if you only have one tail and there is 0,05, shouldn’t the rest be on the other side? why is it then 90%… I know I’m missing a point and I can’t figure it out and it’s so frustrating…

February 23, 2022 at 10:01 pm

The alpha is the total shaded area. So, if the alpha = 0.05, you know that 5% of the distribution is shaded. The number of tails tells you how to divide the shaded areas. Is it all in one region (1-tailed) or do you split the shaded regions in two (2-tailed)?

So, for a one-tailed test with an alpha of 0.05, the 5% shading is all in one tail. If alpha = 0.10, then it’s 10% on one side. If it’s two-tailed, then you need to split that 10% into two–5% in both tails. Hence, the 5% in a one-tailed test is the same as a two-tailed test with an alpha of 0.10 because that test has the same 5% on one side (but there’s another 5% in the other tail).

It’s similar for CIs. However, for CIs, you shade the middle rather than the extremities. I write about that in one my articles about hypothesis testing and confidence intervals .

I’m not sure if I’m answering your question or not.

February 17, 2022 at 1:46 pm

I ran a post hoc Dunnett’s test alpha=0.05 after a significant Anova test in Proc Mixed using SAS. I want to determine if the means for treatment (t1, t2, t3) is significantly less than the means for control (p=pathogen). The code for the dunnett’s test is – LSmeans trt / diff=controll (‘P’) adjust=dunnett CL plot=control; I think the lower bound one tailed test is the correct test to run but I’m not 100% sure. I’m finding conflicting information online. In the output table for the dunnett’s test the mean difference between the control and the treatments is t1=9.8, t2=64.2, and t3=56.5. The control mean estimate is 90.5. The adjusted p-value by treatment is t1(p=0.5734), t2 (p=.0154) and t3(p=.0245). The adjusted lower bound confidence limit in order from t1-t3 is -38.8, 13.4, and 7.9. The adjusted upper bound for all test is infinity. The graphical output for the dunnett’s test in SAS is difficult to understand for those of us who are beginner SAS users. All treatments appear as a vertical line below the the horizontal line for control at 90.5 with t2 and t3 in the shaded area. For treatment 1 the shaded area is above the line for control. Looking at just the output table I would say that t2 and t3 are significantly lower than the control. I guess I would like to know if my interpretation of the outputs is correct that treatments 2 and 3 are statistically significantly lower than the control? Should I have used an upper bound one tailed test instead?

November 10, 2021 at 1:00 am

Thanks Jim. Please help me understand how a two tailed testing can be used to minimize errors in research

July 1, 2021 at 9:19 am

Hi Jim, Thanks for posting such a thorough and well-written explanation. It was extremely useful to clear up some doubts.

May 7, 2021 at 4:27 pm

Hi Jim, I followed your instructions for the Excel add-in. Thank you. I am very new to statistics and sort of enjoy it as I enter week number two in my class. I am to select if three scenarios call for a one or two-tailed test is required and why. The problem is stated:

30% of mole biopsies are unnecessary. Last month at his clinic, 210 out of 634 had benign biopsy results. Is there enough evidence to reject the dermatologist’s claim?

Part two, the wording changes to “more than of 30% of biopsies,” and part three, the wording changes to “less than 30% of biopsies…”

I am not asking for the problem to be solved for me, but I cannot seem to find direction needed. I know the elements i am dealing with are =30%, greater than 30%, and less than 30%. 210 and 634. I just don’t know what to with the information. I can’t seem to find an example of a similar problem to work with.

May 9, 2021 at 9:22 pm

As I detail in this post, a two-tailed test tells you whether an effect exists in either direction. Or, is it different from the null value in either direction. For the first example, the wording suggests you’d need a two-tailed test to determine whether the population proportion is ≠ 30%. Whenever you just need to know ≠, it suggests a two-tailed test because you’re covering both directions.

For part two, because it’s in one direction (greater than), you need a one-tailed test. Same for part three but it’s less than. Look in this blog post to see how you’d construct the null and alternative hypotheses for these cases. Note that you’re working with a proportion rather than the mean, but the principles are the same! Just plug your scenario and the concept of proportion into the wording I use for the hypotheses.

I hope that helps!

April 11, 2021 at 9:30 am

Hello Jim, great website! I am using a statistics program (SPSS) that does NOT compute one-tailed t-tests. I am trying to compare two independent groups and have justifiable reasons why I only care about one direction. Can I do the following? Use SPSS for two-tailed tests to calculate the t & p values. Then report the p-value as p/2 when it is in the predicted direction (e.g , SPSS says p = .04, so I report p = .02), and report the p-value as 1 – (p/2) when it is in the opposite direction (e.g., SPSS says p = .04, so I report p = .98)? If that is incorrect, what do you suggest (hopefully besides changing statistics programs)? Also, if I want to report confidence intervals, I realize that I would only have an upper or lower bound, but can I use the CI’s from SPSS to compute that? Thank you very much!

April 11, 2021 at 5:42 pm

Yes, for p-values, that’s absolutely correct for both cases.

For confidence intervals, if you take one endpoint of a two-side CI, it becomes a one-side bound with half the confidence level.

Consequently, to obtain a one-sided bound with your desired confidence level, you need to take your desired significance level (e.g., 0.05) and double it. Then subtract it from 1. So, if you’re using a significance level of 0.05, double that to 0.10 and then subtract from 1 (1 – 0.10 = 0.90). 90% is the confidence level you want to use for a two-sided test. After obtaining the two-sided CI, use one of the endpoints depending on the direction of your hypothesis (i.e., upper or lower bound). That’s produces the one-sided the bound with the confidence level that you want. For our example, we calculated a 95% one-sided bound.

March 3, 2021 at 8:27 am

Hi Jim. I used the one-tailed(right) statistical test to determine an anomaly in the below problem statement: On a daily basis, I calculate the (mapped_%) in a common field between two tables.

The way I used the t-test is: On any particular day, I calculate the sample_mean, S.D and sample_count (n=30) for the last 30 days including the current day. My null hypothesis, H0 (pop. mean)=95 and H1>95 (alternate hypothesis). So, I calculate the t-stat based on the sample_mean, pop.mean, sample S.D and n. I then choose the t-crit value for 0.05 from my t-ditribution table for dof(n-1). On the current day if my abs.(t-stat)>t-crit, then I reject the null hypothesis and I say the mapped_pct on that day has passed the t-test.

I get some weird results here, where if my mapped_pct is as low as 6%-8% in all the past 30 days, the t-test still gets a “pass” result. Could you help on this? If my hypothesis needs to be changed.

I would basically look for the mapped_pct >95, if it worked on a static trigger. How can I use the t-test effectively in this problem statement?

December 18, 2020 at 8:23 pm

Hello Dr. Jim, I am wondering if there is evidence in one of your books or other source you could provide, which supports that it is OK not to divide alpha level by 2 in one-tailed hypotheses. I need the source for supporting evidence in a Portfolio exercise and couldn’t find one.

I am grateful for your reply and for your statistics knowledge sharing!

November 27, 2020 at 10:31 pm

If I did a one directional F test ANOVA(one tail ) and wanted to calculate a confidence interval for each individual groups (3) mean . Would I use a one tailed or two tailed t , within my confidence interval .

November 29, 2020 at 2:36 am

Hi Bashiru,

F-tests for ANOVA will always be one-tailed for the reasons I discuss in this post. To learn more about, read my post about F-tests in ANOVA .

For the differences between my groups, I would not use t-tests because the family-wise error rate quickly grows out of hand. To learn more about how to compare group means while controlling the familywise error rate, read my post about using post hoc tests with ANOVA . Typically, these are two-side intervals but you’d be able to use one-sided.

November 26, 2020 at 10:51 am

Hi Jim, I had a question about the formulation of the hypotheses. When you want to test if a beta = 1 or a beta = 0. What will be the null hypotheses? I’m having trouble with finding out. Because in most cases beta = 0 is the null hypotheses but in this case you want to test if beta = 0. so i’m having my doubts can it in this case be the alternative hypotheses or is it still the null hypotheses?

Kind regards, Noa

November 27, 2020 at 1:21 am

Typically, the null hypothesis represents no effect or no relationship. As an analyst, you’re hoping that your data have enough evidence to reject the null and favor the alternative.

Assuming you’re referring to beta as in regression coefficients, zero represents no relationship. Consequently, beta = 0 is the null hypothesis.

You might hope that beta = 1, but you don’t usually include that in your alternative hypotheses. The alternative hypothesis usually states that it does not equal no effect. In other words, there is an effect but it doesn’t state what it is.

There are some exceptions to the above but I’m writing about the standard case.

November 22, 2020 at 8:46 am

Your articles are a help to intro to econometrics students. Keep up the good work! More power to you!

November 6, 2020 at 11:25 pm

Hello Jim. Can you help me with these please?

Write the null and alternative hypothesis using a 1-tailed and 2-tailed test for each problem. (In paragraph and symbols)

A teacher wants to know if there is a significant difference in the performance in MAT C313 between her morning and afternoon classes.

It is known that in our university canteen, the average waiting time for a customer to receive and pay for his/her order is 20 minutes. Additional personnel has been added and now the management wants to know if the average waiting time had been reduced.

November 8, 2020 at 12:29 am

I cover how to write the hypotheses for the different types of tests in this post. So, you just need to figure which type of test you need to use. In your case, you want to determine whether the mean waiting time is less than the target value of 20 minutes. That’s a 1-sample t-test because you’re comparing a mean to a target value (20 minutes). You specifically want to determine whether the mean is less than the target value. So, that’s a one-tailed test. And, you’re looking for a mean that is “less than” the target.

So, go to the one-tailed section in the post and look for the hypotheses for the effect being less than. That’s the one with the critical region on the left side of the curve.

Now, you need include your own information. In your case, you’re comparing the sample estimate to a population mean of 20. The 20 minutes is your null hypothesis value. Use the symbol mu μ to represent the population mean.

You put all that together and you get the following:

Null: μ ≥ 20 Alternative: μ 0 to denote the null hypothesis and H 1 or H A to denote the alternative hypothesis if that’s what you been using in class.

October 17, 2020 at 12:11 pm

I was just wondering if you could please help with clarifying what the hypothesises would be for say income for gamblers and, age of gamblers. I am struggling to find which means would be compared.

October 17, 2020 at 7:05 pm

Those are both continuous variables, so you’d use either correlation or regression for them. For both of those analyses, the hypotheses are the following:

Null : The correlation or regression coefficient equals zero (i.e., there is no relationship between the variables) Alternative : The coefficient does not equal zero (i.e., there is a relationship between the variables.)

When the p-value is less than your significance level, you reject the null and conclude that a relationship exists.

October 17, 2020 at 3:05 am

I was ask to choose and justify the reason between a one tailed and two tailed test for dummy variables, how do I do that and what does it mean?

October 17, 2020 at 7:11 pm

I don’t have enough information to answer your question. A dummy variable is also known as an indicator variable, which is a binary variable that indicates the presence or absence of a condition or characteristic. If you’re using this variable in a hypothesis test, I’d presume that you’re using a proportions test, which is based on the binomial distribution for binary data.

Choosing between a one-tailed or two-tailed test depends on subject area issues and, possibly, your research objectives. Typically, use a two-tailed test unless you have a very good reason to use a one-tailed test. To understand when you might use a one-tailed test, read my post about when to use a one-tailed hypothesis test .

October 16, 2020 at 2:07 pm

In your one-tailed example, Minitab describes the hypotheses as “Test of mu = 100 vs > 100”. Any idea why Minitab says the null is “=” rather than “= or less than”? No ASCII character for it?

October 16, 2020 at 4:20 pm

I’m not entirely sure even though I used to work there! I know we had some discussions about how to represent that hypothesis but I don’t recall the exact reasoning. I suspect that it has to do with the conclusions that you can draw. Let’s focus on the failing to reject the null hypothesis. If the test statistic falls in that region (i.e., it is not significant), you fail to reject the null. In this case, all you know is that you have insufficient evidence to say it is different than 100. I’m pretty sure that’s why they use the equal sign because it might as well be one.

Mathematically, I think using ≤ is more accurate, which you can really see when you look at the distribution plots. That’s why I phrase the hypotheses using ≤ or ≥ as needed. However, in terms of the interpretation, the “less than” portion doesn’t really add anything of importance. You can conclude that its equal to 100 or greater than 100, but not less than 100.

October 15, 2020 at 5:46 am

Thank you so much for your timely feedback. It helps a lot

October 14, 2020 at 10:47 am

How can i use one tailed test at 5% alpha on this problem?

A manufacturer of cellular phone batteries claims that when fully charged, the mean life of his product lasts for 26 hours with a standard deviation of 5 hours. Mr X, a regular distributor, randomly picked and tested 35 of the batteries. His test showed that the average life of his sample is 25.5 hours. Is there a significant difference between the average life of all the manufacturer’s batteries and the average battery life of his sample?

October 14, 2020 at 8:22 pm

I don’t think you’d want to use a one-tailed test. The goal is to determine whether the sample is significantly different than the manufacturer’s population average. You’re not saying significantly greater than or less than, which would be a one-tailed test. As phrased, you want a two-tailed test because it can detect a difference in either direct.

It sounds like you need to use a 1-sample t-test to test the mean. During this test, enter 26 as the test mean. The procedure will tell you if the sample mean of 25.5 hours is a significantly different from that test mean. Similarly, you’d need a one variance test to determine whether the sample standard deviation is significantly different from the test value of 5 hours.

For both of these tests, compare the p-value to your alpha of 0.05. If the p-value is less than this value, your results are statistically significant.

September 22, 2020 at 4:16 am

Hi Jim, I didn’t get an idea that when to use two tail test and one tail test. Will you please explain?

September 22, 2020 at 10:05 pm

I have a complete article dedicated to that: When Can I Use One-Tailed Tests .

Basically, start with the assumption that you’ll use a two-tailed test but then consider scenarios where a one-tailed test can be appropriate. I talk about all of that in the article.

If you have questions after reading that, please don’t hesitate to ask!

July 31, 2020 at 12:33 pm

Thank you so so much for this webpage.

I have two scenarios that I need some clarification. I will really appreciate it if you can take a look:

So I have several of materials that I know when they are tested after production. My hypothesis is that the earlier they are tested after production, the higher the mean value I should expect. At the same time, the later they are tested after production, the lower the mean value. Since this is more like a “greater or lesser” situation, I should use one tail. Is that the correct approach?

On the other hand, I have several mix of materials that I don’t know when they are tested after production. I only know the mean values of the test. And I only want to know whether one mean value is truly higher or lower than the other, I guess I want to know if they are only significantly different. Should I use two tail for this? If they are not significantly different, I can judge based on the mean values of test alone. And if they are significantly different, then I will need to do other type of analysis. Also, when I get my P-value for two tail, should I compare it to 0.025 or 0.05 if my confidence level is 0.05?

Thank you so much again.

July 31, 2020 at 11:19 pm

For your first, if you absolutely know that the mean must be lower the later the material is tested, that it cannot be higher, that would be a situation where you can use a one-tailed test. However, if that’s not a certainty, you’re just guessing, use a two-tail test. If you’re measuring different items at the different times, use the independent 2-sample t-test. However, if you’re measuring the same items at two time points, use the paired t-test. If it’s appropriate, using the paired t-test will give you more statistical power because it accounts for the variability between items. For more information, see my post about when it’s ok to use a one-tailed test .

For the mix of materials, use a two-tailed test because the effect truly can go either direction.

Always compare the p-value to your full significance level regardless of whether it’s a one or two-tailed test. Don’t divide the significance level in half.

June 17, 2020 at 2:56 pm

Is it possible that we reach to opposite conclusions if we use a critical value method and p value method Secondly if we perform one tail test and use p vale method to conclude our Ho, then do we need to convert sig value of 2 tail into sig value of one tail. That can be done just by dividing it with 2

June 18, 2020 at 5:17 pm

The p-value method and critical value method will always agree as long as you’re not changing anything about how the methodology.

If you’re using statistical software, you don’t need to make any adjustments. The software will do that for you.

However, if you calculating it by hand, you’ll need to take your significance level and then look in the table for your test statistic for a one-tailed test. For example, you’ll want to look up 5% for a one-tailed test rather than a two-tailed test. That’s not as simple as dividing by two. In this article, I show examples of one-tailed and two-tailed tests for the same degrees of freedom. The t critical value for the two-tailed test is +/- 2.086 while for the one-sided test it is 1.725. It is true that probability associated with those critical values doubles for the one-tailed test (2.5% -> 5%), but the critical value itself is not half (2.086 -> 1.725). Study the first several graphs in this article to see why that is true.

For the p-value, you can take a two-tailed p-value and divide by 2 to determine the one-sided p-value. However, if you’re using statistical software, it does that for you.

June 11, 2020 at 3:46 pm

Hello Jim, if you have the time I’d be grateful if you could shed some clarity on this scenario:

“A researcher believes that aromatherapy can relieve stress but wants to determine whether it can also enhance focus. To test this, the researcher selected a random sample of students to take an exam in which the average score in the general population is 77. Prior to the exam, these students studied individually in a small library room where a lavender scent was present. If students in this group scored significantly above the average score in general population [is this one-tailed or two-tailed hypothesis?], then this was taken as evidence that the lavender scent enhanced focus.”

Thank you for your time if you do decide to respond.

June 11, 2020 at 4:00 pm

It’s unclear from the information provided whether the researchers used a one-tailed or two-tailed test. It could be either. A two-tailed test can detect effects in both directions, so it could definitely detect an average group score above the population score. However, you could also detect that effect using a one-tailed test if it was set up correctly. So, there’s not enough information in what you provided to know for sure. It could be either.

However, that’s irrelevant to answering the question. The tricky part, as I see it, is that you’re not entirely sure about why the scores are higher. Are they higher because the lavender scent increased concentration or are they higher because the subjects have lower stress from the lavender? Or, maybe it’s not even related to the scent but some other characteristic of the room or testing conditions in which they took the test. You just know the scores are higher but not necessarily why they’re higher.

I’d say that, no, it’s not necessarily evidence that the lavender scent enhanced focus. There are competing explanations for why the scores are higher. Also, it would be best do this as an experiment with a control and treatment group where subjects are randomly assigned to either group. That process helps establish causality rather than just correlation and helps rules out competing explanations for why the scores are higher.

By the way, I spend a lot of time on these issues in my Introduction to Statistics ebook .

June 9, 2020 at 1:47 pm

If a left tail test has an alpha value of 0.05 how will you find the value in the table

April 19, 2020 at 10:35 am

Hi Jim, My question is in regards to the results in the table in your example of the one-sample T (Two-Tailed) test. above. What about the P-value? The P-value listed is .018. I assuming that is compared to and alpha of 0.025, correct?

In regression analysis, when I get a test statistic for the predictive variable of -2.099 and a p-value of 0.039. Am I comparing the p-value to an alpha of 0.025 or 0.05? Now if I run a Bootstrap for coefficients analysis, the results say the sig (2-tail) is 0.098. What are the critical values and alpha in this case? I’m trying to reconcile what I am seeing in both tables.

Thanks for your help.

April 20, 2020 at 3:24 am

Hi Marvalisa,

For one-tailed tests, you don’t need to divide alpha in half. If you can tell your software to perform a one-tailed test, it’ll do all the calculations necessary so you don’t need to adjust anything. So, if you’re using an alpha of 0.05 for a one-tailed test and your p-value is 0.04, it is significant. The procedures adjust the p-values automatically and it all works out. So, whether you’re using a one-tailed or two-tailed test, you always compare the p-value to the alpha with no need to adjust anything. The procedure does that for you!

The exception would be if for some reason your software doesn’t allow you to specify that you want to use a one-tailed test instead of a two-tailed test. Then, you divide the p-value from a two-tailed test in half to get the p-value for a one tailed test. You’d still compare it to your original alpha.

For regression, the same thing applies. If you want to use a one-tailed test for a cofficient, just divide the p-value in half if you can’t tell the software that you want a one-tailed test. The default is two-tailed. If your software has the option for one-tailed tests for any procedure, including regression, it’ll adjust the p-value for you. So, in the normal course of things, you won’t need to adjust anything.

March 26, 2020 at 12:00 pm

Hey Jim, for a one-tailed hypothesis test with a .05 confidence level, should I use a 95% confidence interval or a 90% confidence interval? Thanks

March 26, 2020 at 5:05 pm

You should use a one-sided 95% confidence interval. One-sided CIs have either an upper OR lower bound but remains unbounded on the other side.

March 16, 2020 at 4:30 pm

This is not applicable to the subject but… When performing tests of equivalence, we look at the confidence interval of the difference between two groups, and we perform two one-sided t-tests for equivalence..

March 15, 2020 at 7:51 am

Thanks for this illustrative blogpost. I had a question on one of your points though.

By definition of H1 and H0, a two-sided alternate hypothesis is that there is a difference in means between the test and control. Not that anything is ‘better’ or ‘worse’.

Just because we observed a negative result in your example, does not mean we can conclude it’s necessarily worse, but instead just ‘different’.

Therefore while it enables us to spot the fact that there may be differences between test and control, we cannot make claims about directional effects. So I struggle to see why they actually need to be used instead of one-sided tests.

What’s your take on this?

March 16, 2020 at 3:02 am

Hi Dominic,

If you’ll notice, I carefully avoid stating better or worse because in a general sense you’re right. However, given the context of a specific experiment, you can conclude whether a negative value is better or worse. As always in statistics, you have to use your subject-area knowledge to help interpret the results. In some cases, a negative value is a bad result. In other cases, it’s not. Use your subject-area knowledge!

I’m not sure why you think that you can’t make claims about directional effects? Of course you can!

As for why you shouldn’t use one-tailed tests for most cases, read my post When Can I Use One-Tailed Tests . That should answer your questions.

May 10, 2019 at 12:36 pm

Your website is absolutely amazing Jim, you seem like the nicest guy for doing this and I like how there’s no ulterior motive, (I wasn’t automatically signed up for emails or anything when leaving this comment). I study economics and found econometrics really difficult at first, but your website explains it so clearly its been a big asset to my studies, keep up the good work!

May 10, 2019 at 2:12 pm

Thank you so much, Jack. Your kind words mean a lot!

April 26, 2019 at 5:05 am

Hy Jim I really need your help now pls

One-tailed and two- tailed hypothesis, is it the same or twice, half or unrelated pls

April 26, 2019 at 11:41 am

Hi Anthony,

I describe how the hypotheses are different in this post. You’ll find your answers.

February 8, 2019 at 8:00 am

Thank you for your blog Jim, I have a Statistics exam soon and your articles let me understand a lot!

February 8, 2019 at 10:52 am

You’re very welcome! I’m happy to hear that it’s been helpful. Best of luck on your exam!

January 12, 2019 at 7:06 am

Hi Jim, When you say target value is 5. Do you mean to say the population mean is 5 and we are trying to validate it with the help of sample mean 4.1 using Hypo tests ?.. If it is so.. How can we measure a population parameter as 5 when it is almost impossible o measure a population parameter. Please clarify

January 12, 2019 at 6:57 pm

When you set a target for a one-sample test, it’s based on a value that is important to you. It’s not a population parameter or anything like that. The example in this post uses a case where we need parts that are stronger on average than a value of 5. We derive the value of 5 by using our subject area knowledge about what is required for a situation. Given our product knowledge for the hypothetical example, we know it should be 5 or higher. So, we use that in the hypothesis test and determine whether the population mean is greater than that target value.

When you perform a one-sample test, a target value is optional. If you don’t supply a target value, you simply obtain a confidence interval for the range of values that the parameter is likely to fall within. But, sometimes there is meaningful number that you want to test for specifically.

I hope that clarifies the rational behind the target value!

November 15, 2018 at 8:08 am

I understand that in Psychology a one tailed hypothesis is preferred. Is that so

November 15, 2018 at 11:30 am

No, there’s no overall preference for one-tailed hypothesis tests in statistics. That would be a study-by-study decision based on the types of possible effects. For more information about this decision, read my post: When Can I Use One-Tailed Tests?

November 6, 2018 at 1:14 am

I’m grateful to you for the explanations on One tail and Two tail hypothesis test. This opens my knowledge horizon beyond what an average statistics textbook can offer. Please include more examples in future posts. Thanks

November 5, 2018 at 10:20 am

Thank you. I will search it as well.

Stan Alekman

November 4, 2018 at 8:48 pm

Jim, what is the difference between the central and non-central t-distributions w/respect to hypothesis testing?

November 5, 2018 at 10:12 am

Hi Stan, this is something I will need to look into. I know central t-distribution is the common Student t-distribution, but I don’t have experience using non-central t-distributions. There might well be a blog post in that–after I learn more!

November 4, 2018 at 7:42 pm

this is awesome.

Comments and Questions Cancel reply

Statistics Tutorial

Descriptive statistics, inferential statistics, stat reference, statistics - hypothesis testing a mean (left tailed).

A population mean is an average of value a population.

Hypothesis tests are used to check a claim about the size of that population mean.

Hypothesis Testing a Mean

The following steps are used for a hypothesis test:

• Check the conditions
• Define the claims
• Decide the significance level
• Calculate the test statistic

For example:

• Population : Nobel Prize winners
• Category : Age when they received the prize.

And we want to check the claim:

"The average age of Nobel Prize winners when they received the prize is less than 60"

By taking a sample of 30 randomly selected Nobel Prize winners we could find that:

The mean age in the sample (\(\bar{x}\)) is 62.1

The standard deviation of age in the sample (\(s\)) is 13.46

From this sample data we check the claim with the steps below.

1. Checking the Conditions

The conditions for calculating a confidence interval for a proportion are:

• The sample is randomly selected
• The population data is normally distributed
• Sample size is large enough

A moderately large sample size, like 30, is typically large enough.

In the example, the sample size was 30 and it was randomly selected, so the conditions are fulfilled.

Note: Checking if the data is normally distributed can be done with specialized statistical tests.

2. Defining the Claims

We need to define a null hypothesis (\(H_{0}\)) and an alternative hypothesis (\(H_{1}\)) based on the claim we are checking.

The claim was:

In this case, the parameter is the mean age of Nobel Prize winners when they received the prize (\(\mu\)).

The null and alternative hypothesis are then:

Null hypothesis : The average age was 60.

Alternative hypothesis : The average age was less than 60.

Which can be expressed with symbols as:

\(H_{0}\): \(\mu = 60 \)

\(H_{1}\): \(\mu < 60 \)

This is a ' left tailed' test, because the alternative hypothesis claims that the proportion is less than in the null hypothesis.

If the data supports the alternative hypothesis, we reject the null hypothesis and accept the alternative hypothesis.

Advertisement

3. Deciding the Significance Level

The significance level (\(\alpha\)) is the uncertainty we accept when rejecting the null hypothesis in a hypothesis test.

The significance level is a percentage probability of accidentally making the wrong conclusion.

Typical significance levels are:

• \(\alpha = 0.1\) (10%)
• \(\alpha = 0.05\) (5%)
• \(\alpha = 0.01\) (1%)

A lower significance level means that the evidence in the data needs to be stronger to reject the null hypothesis.

There is no "correct" significance level - it only states the uncertainty of the conclusion.

Note: A 5% significance level means that when we reject a null hypothesis:

We expect to reject a true null hypothesis 5 out of 100 times.

4. Calculating the Test Statistic

The test statistic is used to decide the outcome of the hypothesis test.

The test statistic is a standardized value calculated from the sample.

The formula for the test statistic (TS) of a population mean is:

\(\displaystyle \frac{\bar{x} - \mu}{s} \cdot \sqrt{n} \)

\(\bar{x}-\mu\) is the difference between the sample mean (\(\bar{x}\)) and the claimed population mean (\(\mu\)).

\(s\) is the sample standard deviation .

\(n\) is the sample size.

In our example:

The claimed (\(H_{0}\)) population mean (\(\mu\)) was \( 60 \)

The sample mean (\(\bar{x}\)) was \(62.1\)

The sample standard deviation (\(s\)) was \(13.46\)

The sample size (\(n\)) was \(30\)

So the test statistic (TS) is then:

\(\displaystyle \frac{62.1-60}{13.46} \cdot \sqrt{30} = \frac{2.1}{13.46} \cdot \sqrt{30} \approx 0.156 \cdot 5.477 = \underline{0.855}\)

You can also calculate the test statistic using programming language functions:

With Python use the scipy and math libraries to calculate the test statistic.

With R use built-in math and statistics functions to calculate the test statistic.

5. Concluding

There are two main approaches for making the conclusion of a hypothesis test:

• The critical value approach compares the test statistic with the critical value of the significance level.
• The P-value approach compares the P-value of the test statistic and with the significance level.

Note: The two approaches are only different in how they present the conclusion.

The Critical Value Approach

For the critical value approach we need to find the critical value (CV) of the significance level (\(\alpha\)).

For a population mean test, the critical value (CV) is a T-value from a student's t-distribution .

This critical T-value (CV) defines the rejection region for the test.

The rejection region is an area of probability in the tails of the standard normal distribution.

Because the claim is that the population mean is less than 60, the rejection region is in the left tail:

The student's t-distribution is adjusted for the uncertainty from smaller samples.

This adjustment is called degrees of freedom (df), which is the sample size \((n) - 1\)

In this case the degrees of freedom (df) is: \(30 - 1 = \underline{29} \)

Choosing a significance level (\(\alpha\)) of 0.05, or 5%, we can find the critical T-value from a T-table , or with a programming language function:

With Python use the Scipy Stats library t.ppf() function find the T-Value for an \(\alpha\) = 0.05 at 29 degrees of freedom (df).

With R use the built-in qt() function to find the t-value for an \(\alpha\) = 0.05 at 29 degrees of freedom (df).

Using either method we can find that the critical T-Value is \(\approx \underline{-1.699}\)

For a left tailed test we need to check if the test statistic (TS) is smaller than the critical value (CV).

If the test statistic is smaller the critical value, the test statistic is in the rejection region .

When the test statistic is in the rejection region, we reject the null hypothesis (\(H_{0}\)).

Here, the test statistic (TS) was \(\approx \underline{0.855}\) and the critical value was \(\approx \underline{-1.699}\)

Here is an illustration of this test in a graph:

Since the test statistic was bigger than the critical value we keep the null hypothesis.

This means that the sample data does not support the alternative hypothesis.

And we can summarize the conclusion stating:

The sample data does not support the claim that "The average age of Nobel Prize winners when they received the prize is less than 60" at a 5% significance level .

The P-Value Approach

For the P-value approach we need to find the P-value of the test statistic (TS).

If the P-value is smaller than the significance level (\(\alpha\)), we reject the null hypothesis (\(H_{0}\)).

The test statistic was found to be \( \approx \underline{0.855} \)

For a population proportion test, the test statistic is a T-Value from a student's t-distribution .

Because this is a left tailed test, we need to find the P-value of a t-value smaller than 0.855.

The student's t-distribution is adjusted according to degrees of freedom (df), which is the sample size \((30) - 1 = \underline{29}\)

We can find the P-value using a T-table , or with a programming language function:

With Python use the Scipy Stats library t.cdf() function find the P-value of a T-value smaller than 0.855 at 29 degrees of freedom (df):

With R use the built-in pt() function find the P-value of a T-Value smaller than 0.855 at 29 degrees of freedom (df):

Using either method we can find that the P-value is \(\approx \underline{0.800}\)

This tells us that the significance level (\(\alpha\)) would need to be smaller 0.80, or 80%, to reject the null hypothesis.

This P-value is far bigger than any of the common significance levels (10%, 5%, 1%).

So the null hypothesis is kept at all of these significance levels.

The sample data does not support the claim that "The average age of Nobel Prize winners when they received the prize is less than 60" at a 10%, 5%, or 1% significance level .

Calculating a P-Value for a Hypothesis Test with Programming

Many programming languages can calculate the P-value to decide outcome of a hypothesis test.

Using software and programming to calculate statistics is more common for bigger sets of data, as calculating manually becomes difficult.

The P-value calculated here will tell us the lowest possible significance level where the null-hypothesis can be rejected.

With Python use the scipy and math libraries to calculate the P-value for a left tailed hypothesis test for a mean.

Here, the sample size is 30, the sample mean is 62.1, the sample standard deviation is 13.46, and the test is for a mean smaller 60.

With R use built-in math and statistics functions find the P-value for a left tailed hypothesis test for a mean.

Left-Tailed and Two-Tailed Tests

This was an example of a left tailed test, where the alternative hypothesis claimed that parameter is smaller than the null hypothesis claim.

You can check out an equivalent step-by-step guide for other types here:

• Right-Tailed Test
• Two-Tailed Test

COLOR PICKER

Contact Sales

If you want to use W3Schools services as an educational institution, team or enterprise, send us an e-mail: [email protected]

Report Error

If you want to report an error, or if you want to make a suggestion, send us an e-mail: [email protected]

Top Tutorials

Top references, top examples, get certified.

Hypothesis Testing for Means & Proportions

•   1  
• |   2  
• |   3  
• |   4  
• |   5  
• |   6  
• |   7  
• |   8  
• |   9  
• |   10  

Hypothesis Testing: Upper-, Lower, and Two Tailed Tests

Type i and type ii errors.

All Modules

Z score Table

t score Table

The procedure for hypothesis testing is based on the ideas described above. Specifically, we set up competing hypotheses, select a random sample from the population of interest and compute summary statistics. We then determine whether the sample data supports the null or alternative hypotheses. The procedure can be broken down into the following five steps.  

• Step 1. Set up hypotheses and select the level of significance α.

H 0 : Null hypothesis (no change, no difference);  

H 1 : Research hypothesis (investigator's belief); α =0.05

• Step 2. Select the appropriate test statistic.  

The test statistic is a single number that summarizes the sample information.   An example of a test statistic is the Z statistic computed as follows:

When the sample size is small, we will use t statistics (just as we did when constructing confidence intervals for small samples). As we present each scenario, alternative test statistics are provided along with conditions for their appropriate use.

• Step 3.  Set up decision rule.  

The decision rule is a statement that tells under what circumstances to reject the null hypothesis. The decision rule is based on specific values of the test statistic (e.g., reject H 0 if Z > 1.645). The decision rule for a specific test depends on 3 factors: the research or alternative hypothesis, the test statistic and the level of significance. Each is discussed below.

• The decision rule depends on whether an upper-tailed, lower-tailed, or two-tailed test is proposed. In an upper-tailed test the decision rule has investigators reject H 0 if the test statistic is larger than the critical value. In a lower-tailed test the decision rule has investigators reject H 0 if the test statistic is smaller than the critical value.  In a two-tailed test the decision rule has investigators reject H 0 if the test statistic is extreme, either larger than an upper critical value or smaller than a lower critical value.
• The exact form of the test statistic is also important in determining the decision rule. If the test statistic follows the standard normal distribution (Z), then the decision rule will be based on the standard normal distribution. If the test statistic follows the t distribution, then the decision rule will be based on the t distribution. The appropriate critical value will be selected from the t distribution again depending on the specific alternative hypothesis and the level of significance.  
• The third factor is the level of significance. The level of significance which is selected in Step 1 (e.g., α =0.05) dictates the critical value.   For example, in an upper tailed Z test, if α =0.05 then the critical value is Z=1.645.  

The following figures illustrate the rejection regions defined by the decision rule for upper-, lower- and two-tailed Z tests with α=0.05. Notice that the rejection regions are in the upper, lower and both tails of the curves, respectively. The decision rules are written below each figure.

Rejection Region for Upper-Tailed Z Test (H : μ > μ ) with α=0.05 The decision rule is: Reject H if Z 1.645.

α Z 0.10 1.282 0.05 1.645 0.025 1.960 0.010 2.326 0.005 2.576 0.001 3.090 0.0001 3.719 Rejection Region for Lower-Tailed Z Test (H 1 : μ < μ 0 ) with α =0.05 The decision rule is: Reject H 0 if Z < 1.645. a Z 0.10 -1.282 0.05 -1.645 0.025 -1.960 0.010 -2.326 0.005 -2.576 0.001 -3.090 0.0001 -3.719 Rejection Region for Two-Tailed Z Test (H 1 : μ ≠ μ 0 ) with α =0.05 The decision rule is: Reject H 0 if Z < -1.960 or if Z > 1.960. 0.20 1.282 0.10 1.645 0.05 1.960 0.010 2.576 0.001 3.291 0.0001 3.819 The complete table of critical values of Z for upper, lower and two-tailed tests can be found in the table of Z values to the right in "Other Resources." Critical values of t for upper, lower and two-tailed tests can be found in the table of t values in "Other Resources." Step 4. Compute the test statistic.   Here we compute the test statistic by substituting the observed sample data into the test statistic identified in Step 2. Step 5. Conclusion.   The final conclusion is made by comparing the test statistic (which is a summary of the information observed in the sample) to the decision rule. The final conclusion will be either to reject the null hypothesis (because the sample data are very unlikely if the null hypothesis is true) or not to reject the null hypothesis (because the sample data are not very unlikely).   If the null hypothesis is rejected, then an exact significance level is computed to describe the likelihood of observing the sample data assuming that the null hypothesis is true. The exact level of significance is called the p-value and it will be less than the chosen level of significance if we reject H 0 . Statistical computing packages provide exact p-values as part of their standard output for hypothesis tests. In fact, when using a statistical computing package, the steps outlined about can be abbreviated. The hypotheses (step 1) should always be set up in advance of any analysis and the significance criterion should also be determined (e.g., α =0.05). Statistical computing packages will produce the test statistic (usually reporting the test statistic as t) and a p-value. The investigator can then determine statistical significance using the following: If p < α then reject H 0 .   Step 1. Set up hypotheses and determine level of significance H 0 : μ = 191 H 1 : μ > 191                 α =0.05 The research hypothesis is that weights have increased, and therefore an upper tailed test is used. Step 2. Select the appropriate test statistic. Because the sample size is large (n > 30) the appropriate test statistic is Step 3. Set up decision rule.   In this example, we are performing an upper tailed test (H 1 : μ> 191), with a Z test statistic and selected α =0.05.   Reject H 0 if Z > 1.645. We now substitute the sample data into the formula for the test statistic identified in Step 2.   We reject H 0 because 2.38 > 1.645. We have statistically significant evidence at a =0.05, to show that the mean weight in men in 2006 is more than 191 pounds. Because we rejected the null hypothesis, we now approximate the p-value which is the likelihood of observing the sample data if the null hypothesis is true. An alternative definition of the p-value is the smallest level of significance where we can still reject H 0 . In this example, we observed Z=2.38 and for α=0.05, the critical value was 1.645. Because 2.38 exceeded 1.645 we rejected H 0 . In our conclusion we reported a statistically significant increase in mean weight at a 5% level of significance. Using the table of critical values for upper tailed tests, we can approximate the p-value. If we select α=0.025, the critical value is 1.96, and we still reject H 0 because 2.38 > 1.960. If we select α=0.010 the critical value is 2.326, and we still reject H 0 because 2.38 > 2.326. However, if we select α=0.005, the critical value is 2.576, and we cannot reject H 0 because 2.38 < 2.576. Therefore, the smallest α where we still reject H 0 is 0.010. This is the p-value. A statistical computing package would produce a more precise p-value which would be in between 0.005 and 0.010. Here we are approximating the p-value and would report p < 0.010.                   In all tests of hypothesis, there are two types of errors that can be committed. The first is called a Type I error and refers to the situation where we incorrectly reject H 0 when in fact it is true. This is also called a false positive result (as we incorrectly conclude that the research hypothesis is true when in fact it is not). When we run a test of hypothesis and decide to reject H 0 (e.g., because the test statistic exceeds the critical value in an upper tailed test) then either we make a correct decision because the research hypothesis is true or we commit a Type I error. The different conclusions are summarized in the table below. Note that we will never know whether the null hypothesis is really true or false (i.e., we will never know which row of the following table reflects reality). Table - Conclusions in Test of Hypothesis   is True Correct Decision Type I Error is False Type II Error Correct Decision In the first step of the hypothesis test, we select a level of significance, α, and α= P(Type I error). Because we purposely select a small value for α, we control the probability of committing a Type I error. For example, if we select α=0.05, and our test tells us to reject H 0 , then there is a 5% probability that we commit a Type I error. Most investigators are very comfortable with this and are confident when rejecting H 0 that the research hypothesis is true (as it is the more likely scenario when we reject H 0 ). When we run a test of hypothesis and decide not to reject H 0 (e.g., because the test statistic is below the critical value in an upper tailed test) then either we make a correct decision because the null hypothesis is true or we commit a Type II error. Beta (β) represents the probability of a Type II error and is defined as follows: β=P(Type II error) = P(Do not Reject H 0 | H 0 is false). Unfortunately, we cannot choose β to be small (e.g., 0.05) to control the probability of committing a Type II error because β depends on several factors including the sample size, α, and the research hypothesis. When we do not reject H 0 , it may be very likely that we are committing a Type II error (i.e., failing to reject H 0 when in fact it is false). Therefore, when tests are run and the null hypothesis is not rejected we often make a weak concluding statement allowing for the possibility that we might be committing a Type II error. If we do not reject H 0 , we conclude that we do not have significant evidence to show that H 1 is true. We do not conclude that H 0 is true.  The most common reason for a Type II error is a small sample size. return to top | previous page | next page Content ©2017. All Rights Reserved. Date last modified: November 6, 2017. Wayne W. LaMorte, MD, PhD, MPH One Tailed Test or Two in Hypothesis Testing; One Tailed Distribution Area Contents (Click to slip to that section): Alpha levels When should you use either test? One tailed distribution (how to find the area) One tailed test or two in Hypothesis Testing: Overview In hypothesis testing , you are asked to decide if a claim is true or not. For example, if someone says “all Floridian’s have a 50% increased chance of melanoma”, it’s up to you to decide if this claim holds merit. One of the first steps is to look up a z-score , and in order to do that , you need to know if it’s a one tailed test or two . You can figure this out in just a couple of steps. Back to top One tailed test or two in Hypothesis Testing: Steps If you’re lucky enough to be given a picture, you’ll be able to tell if your test is one-tailed or two-tailed by comparing it to the image above. However, most of the time you’re given questions, not pictures. So it’s a matter of deciphering the problem and picking out the important piece of information. You’re basically looking for keywords like equals , more than , or less than . Example question #1: A government official claims that the dropout rate for local schools is 25% . Last year, 190 out of 603 students dropped out. Is there enough evidence to reject the government official’s claim? Example question #2: A government official claims that the dropout rate for local schools is less than 25%. Last year, 190 out of 603 students dropped out. Is there enough evidence to reject the government official’s claim? Example question #3: A government official claims that the dropout rate for local schools is greater than 25%. Last year, 190 out of 603 students dropped out. Is there enough evidence to reject the government official’s claim? Step 1: Read the question. Step 2: Rephrase the claim in the question with an equation. In example question #1, Drop out rate = 25% In example question #2, Drop out rate < 25% In example question #3, Drop out rate > 25%. Step 3: If step 2 has an equals sign in it, this is a two-tailed test. If it has > or < it is a one-tailed test. Like the explanation? Check out the Statistics How To Handbook , which has hundreds of easy to understand definitions and examples, just like this one! Back to top One Tailed Test or Two: Onto some more technical stuff The above should have given you a brief overview of the differences between one-tailed tests and two-tailed tests. For the very beginning of your stats class, that’s probably all the information you need to get by. But once you hit ANOVA and regression analysis , things get a little more challenging. 1. Alpha levels Alpha levels (sometimes just called “significance levels”) are used in hypothesis tests ; it is the probability of making the wrong decision when the null hypothesis is true. A one-tailed test has the entire 5% of the alpha level in one tail (in either the left, or the right tail). A two-tailed test splits your alpha level in half (as in the image to the left). Let’s say you’re working with the standard alpha level of 0.5 (5%). A two tailed test will have half of this (2.5%) in each tail. Very simply, the hypothesis test might go like this: A null hypothesis might state that the mean = x . You’re testing if the mean is way above this or way below. You run a t-test , which churns out a t-statistic . If this test statistic falls in the top 2.5% or bottom 2.5% of its probability distribution (in this case, the t-distribution ), you would reject the null hypothesis . The “cut off” areas created by your alpha levels are called rejection regions . It’s where you would reject the null hypothesis, if your test statistic happens to fall into one of those rejection areas. The terms “one tailed” and “two tailed” can more precisely be defined as referring to where your rejection regions are located. Back to top A one-tailed test is where you are only interested in one direction. If a mean is x, you might want to know if a set of results is more than x or less than x. A one-tailed test is more powerful than a two-tailed test, as you aren’t considering an effect in the opposite direction. Next : Left tailed test or right tailed test? Back to top 3. When Should You Use a One-Tailed Test? In the above examples, you were given specific wording like “greater than” or “less than.” Sometimes you, the researcher, do not have this information and you have to choose the test. For example, you develop a drug which you think is just as effective as a drug already on the market (it also happens to be cheaper). You could run a two-tailed test (to test that it is more effective and to also check that it is less effective). But you don’t really care about it being more effective, just that it isn’t any less effective (after all, your drug is cheaper). You can run a one-tailed test to check that your drug is at least as effective as the existing drug. On the other hand, it would be inappropriate (and perhaps, unethical) to run a one-tailed test for this scenario in the opposite direction (i.e. to show the drug is more effective). This sounds reasonable until you consider there may be certain circumstances where the drug is less effective. If you fail to test for that, your research will be useless. Consider both directions when deciding if you should run a one tailed test or two. If you can skip one tail and it’s not irresponsible or unethical to do so, then you can run a one-tailed test. Back to top One tailed Test or Two: How to find the area of a one-tailed distribution: Steps There are a few ways to find the area under a one tailed distribution curve. The easiest, by far, is looking up the value in a table like the z-table . A z-table gives you percentages, which represent the area under a curve . For example, a table value of 0.5000 is 50% of the area and 0.2000 is 20% of the area. If you are looking for other area problems*, see the normal distribution curve index . The index lists seven possible types of area, including two tailed, one tailed, and areas to the left and right of z. *You can also calculate areas with integral calculus . See The Area Problem . Note : In order to use a z-table , you need to split your z-value up into decimal places (e.g. tenths and hundredths). For example, if you are asked to find the area in a one tailed distribution with a z-value of 0.21, split this into tenths (0.2) and hundredths (0.01). One tailed distribution: Steps for finding the area in a z-table Step 1: Look up your z-score in the z-table . Looking up the value means finding the intersection of your two decimals (see note above). For example, if you are asked to find the area in a one tailed distribution to the left of z = -0.46, look up 0.46 in the table (note: ignore negative values. If you have a negative value, use its absolute value ). The table below shows that the value in the intersection for 0.46 is .1772. This figure was obtained by looking up 0.4 in the left hand column and 0.06 in the top row. z 0.00 0.01 0.02 0.03 0.04 0.05 0.06 0.07 0.08 0.09 0.0 0.0000 0.0040 0.0080 0.0120 0.0160 0.0199 0.0239 0.0279 0.0319 0.0359 0.1 0.0398 0.0438 0.0478 0.0517 0.0557 0.0596 0.0636 0.0675 0.0714 0.0753 0.2 0.0793 0.0832 0.0871 0.0910 0.0948 0.0987 0.1026 0.1064 0.1103 0.1141 0.3 0.1179 0.1217 0.1255 0.1293 0.1331 0.1368 0.1406 0.1443 0.1480 0.1517 0.4 0.1554 0.1591 0.1628 0.1664 0.1700 0.1736 0.1772 0.1808 0.1844 0.1879 0.5 0.1915 0.1950 0.1985 0.2019 0.2054 0.2088 0.2123 0.2157 0.2190 0.2224 Step 2: Take the area you just found in step 2 and add .500. That’s because the area in the right-hand z-table is the area between the mean and the z-score. You want the entire area up to that point, so: .5000 + .1772 = .6772. Step 3: Subtract from 1 to get the tail area: 1 – .6772 = 0.3228. That’s it! One Tailed Test or Two: References Gonick, L. (1993). The Cartoon Guide to Statistics . HarperPerennial. Heath, D. (2002). An Introduction to Experimental Design and Statistics for Biology. CRC Press. IDRE: FAQ: What are the differences between one-tailed and two-tailed tests? Retrieved May 27, 2018 from: https://stats.idre.ucla.edu/other/mult-pkg/faq/general/faq-what-are-the-differences-between-one-tailed-and-two-tailed-tests/ User Preferences Content preview. Arcu felis bibendum ut tristique et egestas quis: Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris Duis aute irure dolor in reprehenderit in voluptate Excepteur sint occaecat cupidatat non proident Keyboard Shortcuts S.3.1 hypothesis testing (critical value approach). The critical value approach involves determining "likely" or "unlikely" by determining whether or not the observed test statistic is more extreme than would be expected if the null hypothesis were true. That is, it entails comparing the observed test statistic to some cutoff value, called the " critical value ." If the test statistic is more extreme than the critical value, then the null hypothesis is rejected in favor of the alternative hypothesis. If the test statistic is not as extreme as the critical value, then the null hypothesis is not rejected. Specifically, the four steps involved in using the critical value approach to conducting any hypothesis test are: Specify the null and alternative hypotheses. Using the sample data and assuming the null hypothesis is true, calculate the value of the test statistic. To conduct the hypothesis test for the population mean μ , we use the t -statistic \(t^*=\frac{\bar{x}-\mu}{s/\sqrt{n}}\) which follows a t -distribution with n - 1 degrees of freedom. Determine the critical value by finding the value of the known distribution of the test statistic such that the probability of making a Type I error — which is denoted \(\alpha\) (greek letter "alpha") and is called the " significance level of the test " — is small (typically 0.01, 0.05, or 0.10). Compare the test statistic to the critical value. If the test statistic is more extreme in the direction of the alternative than the critical value, reject the null hypothesis in favor of the alternative hypothesis. If the test statistic is less extreme than the critical value, do not reject the null hypothesis. Example S.3.1.1 Mean gpa section  . In our example concerning the mean grade point average, suppose we take a random sample of n = 15 students majoring in mathematics. Since n = 15, our test statistic t * has n - 1 = 14 degrees of freedom. Also, suppose we set our significance level α at 0.05 so that we have only a 5% chance of making a Type I error. Right-Tailed The critical value for conducting the right-tailed test H 0 : μ = 3 versus H A : μ > 3 is the t -value, denoted t \(\alpha\) , n - 1 , such that the probability to the right of it is \(\alpha\). It can be shown using either statistical software or a t -table that the critical value t 0.05,14 is 1.7613. That is, we would reject the null hypothesis H 0 : μ = 3 in favor of the alternative hypothesis H A : μ > 3 if the test statistic t * is greater than 1.7613. Visually, the rejection region is shaded red in the graph. Left-Tailed The critical value for conducting the left-tailed test H 0 : μ = 3 versus H A : μ < 3 is the t -value, denoted -t ( \(\alpha\) , n - 1) , such that the probability to the left of it is \(\alpha\). It can be shown using either statistical software or a t -table that the critical value -t 0.05,14 is -1.7613. That is, we would reject the null hypothesis H 0 : μ = 3 in favor of the alternative hypothesis H A : μ < 3 if the test statistic t * is less than -1.7613. Visually, the rejection region is shaded red in the graph. There are two critical values for the two-tailed test H 0 : μ = 3 versus H A : μ ≠ 3 — one for the left-tail denoted -t ( \(\alpha\) / 2, n - 1) and one for the right-tail denoted t ( \(\alpha\) / 2, n - 1) . The value - t ( \(\alpha\) /2, n - 1) is the t -value such that the probability to the left of it is \(\alpha\)/2, and the value t ( \(\alpha\) /2, n - 1) is the t -value such that the probability to the right of it is \(\alpha\)/2. It can be shown using either statistical software or a t -table that the critical value -t 0.025,14 is -2.1448 and the critical value t 0.025,14 is 2.1448. That is, we would reject the null hypothesis H 0 : μ = 3 in favor of the alternative hypothesis H A : μ ≠ 3 if the test statistic t * is less than -2.1448 or greater than 2.1448. Visually, the rejection region is shaded red in the graph. Statistics Resources Excel - Tutorials Basic Probability Rules Single Event Probability Complement Rule Intersections & Unions Compound Events Levels of Measurement Independent and Dependent Variables Entering Data Central Tendency Data and Tests Displaying Data Discussing Statistics In-text SEM and Confidence Intervals Two-Way Frequency Tables Empirical Rule Finding Probability Accessing SPSS Chart and Graphs Frequency Table and Distribution Descriptive Statistics Converting Raw Scores to Z-Scores Converting Z-scores to t-scores Split File/Split Output Partial Eta Squared Downloading and Installing G*Power: Windows/PC Correlation Testing Parametric Assumptions One-Way ANOVA Two-Way ANOVA Repeated Measures ANOVA Goodness-of-Fit Test of Association Pearson's r Point Biserial Mediation and Moderation Simple Linear Regression Multiple Linear Regression Binomial Logistic Regression Multinomial Logistic Regression Independent Samples T-test Dependent Samples T-test Testing Assumptions T-tests using SPSS T-Test Practice Predictive Analytics This link opens in a new window Quantitative Research Questions Null & Alternative Hypotheses One-Tail vs. Two-Tail Alpha & Beta Associated Probability Decision Rule Statement of Conclusion Statistics Group Sessions One-Tail vs Two-Tail Tests Two-tailed Test When testing a hypothesis, you must determine if it is a one-tailed or a two-tailed test. The most common format is a two-tailed test, meaning the critical region is located in both tails of the distribution. This is also referred to as a non-directional hypothesis. This type of test is associated with a "neutral" alternative hypothesis. Here are some examples: There is a difference between the scores. The groups are not equal . There is a relationship between the variables. One-tailed Test The alternative option is a one-tailed test. As the name implies, the critical region lies in only one tail of the distribution. This is also called a directional  hypothesis. The image below shows a right-tailed test. A left-tailed test would be another type of one-tailed test. This type of test is associated with a more specific alternative claim. Here are some examples: One group is higher than the other. There is a decrease in performance. Group A performs worse than Group B. << Previous: Null & Alternative Hypotheses Next: Alpha & Beta >> Last Updated: Jul 16, 2024 11:19 AM URL: https://resources.nu.edu/statsresources school Campus Bookshelves menu_book Bookshelves perm_media Learning Objects login Login how_to_reg Request Instructor Account hub Instructor Commons Margin Size Download Page (PDF) Download Full Book (PDF) Periodic Table Physics Constants Scientific Calculator Reference & Cite Tools expand_more Readability selected template will load here This action is not available. 8.2: Type I and II Errors Last updated Save as PDF Page ID 24056 Rachel Webb Portland State University \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \) \( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) ( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\) \( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\) \( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\) \( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vectorC}[1]{\textbf{#1}} \) \( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \) \( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \) \( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \) How do you quantify really small? Is 5% or 10% or 15% really small? How do you decide? That depends on your field of study and the importance of the situation. Is this a pilot study? Is someone’s life at risk? Would you lose your job? Most industry standards use 5% as the cutoff point for how small is small enough, but 1%, 5% and 10% are frequently used depending on what the situation calls for. Now, how small is small enough? To answer that, you really want to know the types of errors you can make in hypothesis testing. The first error is if you say that H 0 is false, when in fact it is true. This means you reject H 0 when H 0 was true. The second error is if you say that H 0 is true, when in fact it is false. This means you fail to reject H 0 when H 0 is false. Figure 8-4 shows that if we “Reject H 0 ” when H 0 is actually true, we are committing a type I error. The probability of committing a type I error is the Greek letter \(\alpha\), pronounced alpha. This can be controlled by the researcher by choosing a specific level of significance \(\alpha\). Figure 8-4 shows that if we “Do Not Reject H 0 ” when H 0 is actually false, we are committing a type II error. The probability of committing a type II error is denoted with the Greek letter β, pronounced beta. When we increase the sample size this will reduce β. The power of a test is 1 – β. A jury trial is about to take place to decide if a person is guilty of committing murder. The hypotheses for this situation would be: \(H_0\): The defendant is innocent \(H_1\): The defendant is not innocent The jury has two possible decisions to make, either acquit or convict the person on trial, based on the evidence that is presented. There are two possible ways that the jury could make a mistake. They could convict an innocent person or they could let a guilty person go free. Both are bad news, but if the death penalty was sentenced to the convicted person, the justice system could be killing an innocent person. If a murderer is let go without enough evidence to convict them then they could possibly murder again. In statistics we call these two types of mistakes a type I and II error. Figure 8-5 is a diagram to see the four possible jury decisions and two errors. Type I Error is rejecting H 0 when H 0 is true, and Type II Error is failing to reject H 0 when H 0 is false. Since these are the only two possible errors, one can define the probabilities attached to each error. \(\alpha\) = P(Type I Error) = P(Rejecting H 0 | H 0 is true) β = P(Type II Error) = P(Failing to reject H 0 | H 0 is false) An investment company wants to build a new food cart. They know from experience that food carts are successful if they have on average more than 100 people a day walk by the location. They have a potential site to build on, but before they begin, they want to see if they have enough foot traffic. They observe how many people walk by the site every day over a month. They will build if there is more than an average of 100 people who walk by the site each day. In simple terms, explain what the type I & II errors would be using context from the problem. The hypotheses are: H 0 : μ = 100 and H 1 : μ > 100. Sometimes it is helpful to use words next to your hypotheses instead of the formal symbols H 0 : μ ≤ 100 (Do not build) H 1 : μ > 100 (Build). A type I error would be to reject the null when in fact it is true. Take your finger and cover up the null hypothesis (our decision is to reject the null), then what is showing? The alternative hypothesis is what action we take. If we reject H 0 then we would build the new food cart. However, H 0 was actually true, which means that the mean was less than or equal to 100 people walking by. In more simple terms, this would mean that our evidence showed that we have enough foot traffic to support the food cart. Once we build, though, there was not on average more than 100 people that walk by and the food cart may fail. A type II error would be to fail to reject the null when in fact the null is false. Evidence shows that we should not build on the site, but this actually would have been a prime location to build on. The missed opportunity of a type II error is not as bad as possibly losing thousands of dollars on a bad investment. What is more severe of an error is dependent on what side of the desk you are sitting on. For instance, if a hypothesis is about miles per gallon for a new car the hypotheses may be set up differently depending on if you are buying the car or selling the car. For this course, the claim will be stated in the problem and always set up the hypotheses to match the stated claim. In general, the research question should be set up as some type of change in the alternative hypothesis. Controlling for Type I Error The significance level used by the researcher should be picked prior to collection and analyzing data. This is called “a priori,” versus picking α after you have done your analysis which is called “post hoc.” When deciding on what significance level to pick, one needs to look at the severity of the consequences of the type I and type II errors. For example, if the type I error may cause the loss of life or large amounts of money the researcher would want to set \(\alpha\) low. Controlling for Type II Error The power of a test is the complement of a type II error or correctly rejecting a false null hypothesis. You can increase the power of the test and hence decrease the type II error by increasing the sample size. Similar to confidence intervals, where we can reduce our margin of error when we increase the sample size. In general, we would like to have a high confidence level and a high power for our hypothesis tests. When you increase your confidence level, then in turn the power of the test will decrease. Calculating the probability of a type II error is a little more difficult and it is a conditional probability based on the researcher’s hypotheses and is not discussed in this course. “‘That's right!’ shouted Vroomfondel, ‘we demand rigidly defined areas of doubt and uncertainty!’” (Adams, 2002) Visualizing \(\alpha\) and β If \(\alpha\) increases that means the chances of making a type I error will increase. It is more likely that a type I error will occur. It makes sense that you are less likely to make type II errors, only because you will be rejecting H 0 more often. You will be failing to reject H 0 less, and therefore, the chance of making a type II error will decrease. Thus, as α increases, β will decrease, and vice versa. That makes them seem like complements, but they are not complements. Consider one more factor – sample size. Consider if you have a larger sample that is representative of the population, then it makes sense that you have more accuracy than with a smaller sample. Think of it this way, which would you trust more, a sample mean of 890 if you had a sample size of 35 or sample size of 350 (assuming a representative sample)? Of course, the 350 because there are more data points and so more accuracy. If you are more accurate, then there is less chance that you will make any error. By increasing the sample size of a representative sample, you decrease β. For a constant sample size, n , if \(\alpha\) increases, β decreases. For a constant significance level, \(\alpha\), if n increases, β decreases. When the sample size becomes large, point estimates become more precise and any real differences in the mean and null value become easier to detect and recognize. Even a very small difference would likely be detected if we took a large enough sample size. Sometimes researchers will take such a large sample size that even the slightest difference is detected. While we still say that difference is statistically significant, it might not be practically significant. Statistically significant differences are sometimes so minor that they are not practically relevant. This is especially important to research: if we conduct a study, we want to focus on finding a meaningful result. We do not want to spend lots of money finding results that hold no practical value. The role of a statistician in conducting a study often includes planning the size of the study. The statistician might first consult experts or scientific literature to learn what would be the smallest meaningful difference from the null value. They also would obtain some reasonable estimate for the standard deviation. With these important pieces of information, they would choose a sufficiently large sample size so that the power for the meaningful difference is perhaps 80% or 90%. While larger sample sizes may still be used, the statistician might advise against using them in some cases, especially in sensitive areas of research. If we look at the following two sampling distributions in Figure 8-6, the one on the left represents the sampling distribution for the true unknown mean. The curve on the right represents the sampling distribution based on the hypotheses the researcher is making. Do you remember the difference between a sampling distribution, the distribution of a sample, and the distribution of the population? Revisit the Central Limit Theorem in Chapter 6 if needed. If we start with \(\alpha\) = 0.05, the critical value is represented by the vertical green line at \(z_{\alpha}\) = 1.96. Then the blue shaded area to the right of this line represents \(\alpha\). The area under the curve to the left of \(z_{\alpha / 2}\) = 1.96 based on the researcher’s claim would represent β. If we were to change \(\alpha\) from 0.05 to 0.01 then we get a critical value of \(z_{\alpha / 2}\) = 2.576. Note that when \(\alpha\) decreases, then β increases which means your power 1 – β decreases. See Figure 8-7. This text does not go over how to calculate β. You will need to be able to write out a sentence interpreting either the type I or II errors given a set of hypotheses. You also need to know the relationship between \(\alpha\), β, confidence level, and power. Hypothesis tests are not flawless, since we can make a wrong decision in statistical hypothesis tests based on the data. For example, in the court system, innocent people are sometimes wrongly convicted and the guilty sometimes walk free, or diagnostic tests that have false negatives or false positives. However, the difference is that in statistical hypothesis tests, we have the tools necessary to quantify how often we make such errors. A type I Error is rejecting the null hypothesis when H 0 is actually true. A type II Error is failing to reject the null hypothesis when the alternative is actually true (H 0 is false). We use the symbols \(\alpha\) = P(Type I Error) and β = P(Type II Error). The critical value is a cutoff point on the horizontal axis of the sampling distribution that you can compare your test statistic to see if you should reject the null hypothesis. For a left-tailed test the critical value will always be on the left side of the sampling distribution, the right-tailed test will always be on the right side, and a two-tailed test will be on both tails. Use technology to find the critical values. Most of the time in this course the shortcut menus that we use will give you the critical values as part of the output. 8.2.1 Finding Critical Values A researcher decides they want to have a 5% chance of making a type I error so they set α = 0.05. What z-score would represent that 5% area? It would depend on if the hypotheses were a left-tailed, two-tailed or right-tailed test. This zscore is called a critical value. Figure 8-8 shows examples of critical values for the three possible sets of hypotheses. Two-tailed Test If we are doing a two-tailed test then the \(\alpha\) = 5% area gets divided into both tails. We denote these critical values \(z_{\alpha / 2}\) and \(z_{1-\alpha / 2}\). When the sample data finds a z-score ( test statistic ) that is either less than or equal to \(z_{\alpha / 2}\) or greater than or equal to \(z_{1-\alpha / 2}\) then we would reject H 0 . The area to the left of the critical value \(z_{\alpha / 2}\) and to the right of the critical value \(z_{1-\alpha / 2}\) is called the critical or rejection region. See Figure 8-9. When \(\alpha\) = 0.05 then the critical values \(z_{\alpha / 2}\) and \(z_{1-\alpha / 2}\) are found using the following technology. Excel: \(z_{\alpha / 2}\) =NORM.S.INV(0.025) = –1.96 and \(z_{1-\alpha / 2}\) =NORM.S.INV(0.975) = 1.96 TI-Calculator: \(z_{\alpha / 2}\) = invNorm(0.025,0,1) = –1.96 and \(z_{1-\alpha / 2}\) = invNorm(0.975,0,1) = 1.96 Since the normal distribution is symmetric, you only need to find one side’s z-score and we usually represent the critical values as ± \(z_{\alpha / 2}\). Most of the time we will be finding a probability (p-value) instead of the critical values. The p-value and critical values are related and tell the same information so it is important to know what a critical value represents. Right-tailed Test If we are doing a right-tailed test then the \(\alpha\) = 5% area goes into the right tail. We denote this critical value \(z_{1-\alpha}\). When the sample data finds a z-score more than \(z_{1-\alpha}\) then we would reject H 0 , reject H 0 if the test statistic is ≥ \(z_{1-\alpha}\). The area to the right of the critical value \(z_{1-\alpha}\) is called the critical region. See Figure 8-10. Figure 8-10 When \(\alpha\) = 0.05 then the critical value \(z_{1-\alpha}\) is found using the following technology. Excel: \(z_{1-\alpha}\) =NORM.S.INV(0.95) = 1.645 Figure 8-10 TI-Calculator: \(z_{1-\alpha}\) = invNorm(0.95,0,1) = 1.645 Left-tailed Test If we are doing a left-tailed test then the \(\alpha\) = 5% area goes into the left tail. If the sampling distribution is a normal distribution then we can use the inverse normal function in Excel or calculator to find the corresponding z-score. We denote this critical value \(z_{\alpha}\). When the sample data finds a z-score less than \(z_{\alpha}\) then we would reject H0, reject Ho if the test statistic is ≤ \(z_{\alpha}\). The area to the left of the critical value \(z_{\alpha}\) is called the critical region. See Figure 8-11. Figure 8-11 When \(\alpha\) = 0.05 then the critical value \(z_{\alpha}\) is found using the following technology. Excel: \(z_{\alpha}\) =NORM.S.INV(0.05) = –1.645 TI-Calculator: \(z_{\alpha}\) = invNorm(0.05,0,1) = –1.645 The Claim and Summary The wording on the summary statement changes depending on which hypothesis the researcher claims to be true. We really should always be setting up the claim in the alternative hypothesis since most of the time we are collecting evidence to show that a change has occurred, but occasionally a textbook will have the claim in the null hypothesis. Do not use the phrase “accept H 0 ” since this implies that H0 is true. The lack of evidence is not evidence of nothing. There were only two possible correct answers for the decision step. i. Reject H 0 ii. Fail to reject H 0 Caution! If we fail to reject the null this does not mean that there was no change, we just do not have any evidence that change has occurred. The absence of evidence is not evidence of absence. On the other hand, we need to be careful when we reject the null hypothesis we have not proved that there is change. When we reject the null hypothesis, there is only evidence that a change has occurred. Our evidence could have been false and lead to an incorrect decision. If we use the phrase, “accept H 0 ” this implies that H 0 was true, but we just do not have evidence that it is false. Hence you will be marked incorrect for your decision if you use accept H 0 , use instead “fail to reject H 0 ” or “do not reject H 0 .” Hypothesis Testing: How to Know What Type of Tail we Have? One question that typically hunts students of basic statistics when attempting to solve a hypothesis testing question, be it from a homework or a test, is how to assess what type of tail a hypothesis test has. The problem of determining the type of tail is simply reduced to the correct specification of the null and alternative hypothesis. One has correctly determined the hypotheses for a test, the problem of knowing what type of tail is the correct one (right-tailed, left-tailed or two-tailed) is simple. In order to see the type of tail, we need to look at the alternative hypothesis. If the sign in the alternative hypothesis is "<", then we have a left-tailed test. Or if the sign in the alternative hypothesis is ">", then we have a right-tailed test. Or, on the other hand, if sign in the alternative hypothesis is "≠", then we have a two-tailed test. LET US CONSIDER THE FOLLOWING EXAMPLE : Assume that a simple random sample of the weights of 19 green M&Ms has a mean of 0.8635 grams, and also assume that the population standard deviation \(\sigma\) is known to be 0.0565 g. Let us use a 0.05 significance level to test the claim that the mean weight of all green M&Ms is equal to 0.8535 g, which is the mean weight required so that M&Ms have the weight printed on the package label. Do green M&Ms appear to have weights consistent with the package label? This is how we solve it We want to test the following null and alternative hypotheses \[\begin{align}{{H}_{0}}:\mu {=} {0.8535}\, \\ {{H}_{A}}:\mu {\ne} {0.8535} \\ \end{align}\] Given that the population standard deviation is known, with \(\sigma = 0.0565\) we use the normal distribution. The z-statistic is calculated as \[z =\frac{\bar{X}-\mu }{\sigma / \sqrt{n}}\] We know that this is two-tailed z-test (since the sign in the alternative hypothesis is "≠"). The z-statistics is computed by the following formula: \[z =\frac{\bar{X}-\mu }{\sigma /\sqrt{n}}=\frac{{0.8635}-0.8535}{0.0565 /\sqrt{19}}={0.7715}\] The critical value for \(\alpha = 0.05\) for this two-tailed test found to be \(z_{c} = {1.96}\). The rejection region is corresponds to \[R=\left\{ z:\,\,\,|z|>{1.96} \right\}\] Since \(|z| = 0.7715 {<} z_c = 1.96\), then we fail to reject the null hypothesis H 0 . Thus, we don't have enough evidence to reject the claim green M&Ms appear to have weights consistent with the package label. log in to your account Reset password. Statistics Examples Terms ( Premium ) DO NOT SELL MY INFO Mathway © 2024 Please ensure that your password is at least 8 characters and contains each of the following: a special character: @$#!%*?& 229 IMAGES Everything You Need To Know about Hypothesis Testing PPT Left tailed hypothesis test calculator Statistics Statistics Hypothesis Testing Proportion p Left Tailed P-Value Method VIDEO 1 tailed and 2 tailed Hypothesis One tailed hypothesis and two tailed hypothesis One tailed hypothesis #psychology#statistics #ugcnet #netpsychology Hypothesis Testing & Two-tailed and One-tailed Test (tagalog and basic) Hypothesis Testing for Population Mean (Large sample, Z test) (Hindi/Urdu) Hypothesis Testing "40% of U.S. cell phone owners use their phone for most of their online browsing" COMMENTS How to Identify a Left Tailed Test vs. a Right Tailed Test This tutorial explains how to identify whether a hypothesis test is a left tailed test or a right tailed test in statistics. How to Identify a Left Tailed Test vs. a Right Tailed Test This tutorial explains how to identify whether a hypothesis test is a left tailed test or a right tailed test in statistics. One-Tailed and Two-Tailed Hypothesis Tests Explained Learn how the choice between one and two-tailed hypothesis tests impacts the detectable effects, statistical power, and potential errors. Left Tailed Test or Right Tailed Test ? How to Decide Learn how to choose between left tailed test or right tailed test for your hypothesis testing. Follow easy steps and watch a video. Get online help. Determining if a Hypothesis Test is Left Tailed, Right Tailed, or Two This video explains how to determine the type of hypotheses test. Statistics This is a ' left tailed' test, because the alternative hypothesis claims that the proportion is less than in the null hypothesis. If the data supports the alternative hypothesis, we reject the null hypothesis and accept the alternative hypothesis. Hypothesis Testing: Upper-, Lower, and Two Tailed Tests Hypothesis Testing: Upper-, Lower, and Two Tailed Tests The procedure for hypothesis testing is based on the ideas described above. Specifically, we set up competing hypotheses, select a random sample from the population of interest and compute summary statistics. We then determine whether the sample data supports the null or alternative hypotheses. The procedure can be broken down into the ... 8.4: Tails of a test More specifically, you interpret a one-tailed result in the opposite direction as acceptance of the null — you cannot, after the fact, change your mind and start speaking about "statistically significant differences" if you had specified a one-tailed hypothesis and the results showed differences in the opposite direction. One Tailed Test or Two in Hypothesis Testing: How ... How to figure out if you have a one tailed test or two in hypothesis testing. How to find the area in a one tailed distribution. 11.4: One- and Two-Tailed Tests By contrast, the null hypothesis for the one-tailed test is π ≤ 0.5 π ≤ 0.5. Accordingly, we reject the two-tailed hypothesis if the sample proportion deviates greatly from 0.5 0.5 in either direction. The one-tailed hypothesis is rejected only if the sample proportion is much greater than 0.5 0.5. 8.1.5: Additional Information on Hypothesis Tests When you calculate the p p -value and draw the picture, the p p -value is the area in the left tail, the right tail, or split evenly between the two tails. For this reason, we call the hypothesis test left, right, or two tailed. Hypothesis testing: One-tailed and two-tailed tests The alternate hypothesis for a two-sided t-test would simply state that the mean blood pressure for the medication group is different than the placebo group, but it wouldn't specify if medication would raise or lower the mean blood pressure. Typically, researchers choose to use two-sided t-tests, since they usually don't know how a ... S.3.1 Hypothesis Testing (Critical Value Approach) The critical value for conducting the left-tailed test H0 : μ = 3 versus HA : μ < 3 is the t -value, denoted -t(α, n - 1), such that the probability to the left of it is α. It can be shown using either statistical software or a t -table that the critical value -t0.05,14 is -1.7613. That is, we would reject the null hypothesis H0 : μ = 3 in ... One-Tailed vs Two-Tailed Hypothesis Tests This video explains the differences between one-tailed (right and left) and two-tailed hypothesis tests (directional vs. non-directional)proportion (p) temp... One-Tail vs. Two-Tail The alternative option is a one-tailed test. As the name implies, the critical region lies in only one tail of the distribution. This is also called a directional hypothesis. The image below shows a right-tailed test. A left-tailed test would be another type of one-tailed test. PDF One-tailed Test or Two-tailed Test And then, by comparing test statistic value with the critical value or whether statistic value falls in the critical region, we make a conclusion either to reject the null hypothesis or to fail to reject the null hypothesis. How can we tell whether it is a one-tailed or a two-tailed test? It depends on the original claim in the question. 8.2: Type I and II Errors For a left-tailed test the critical value will always be on the left side of the sampling distribution, the right-tailed test will always be on the right side, and a two-tailed test will be on both tails. Use technology to find the critical values. Hypothesis Testing: How to Know What Type of Tail we Have? The problem of determining the type of tail is simply reduced to the correct specification of the null and alternative hypothesis. One has correctly determined the hypotheses for a test, the problem of knowing what type of tail is the correct one (right-tailed, left-tailed or two-tailed) is simple. Khan Academy Khanmigo is now free for all US educators! Plan lessons, develop exit tickets, and so much more with our AI teaching assistant. Get it now! Statistics Examples Alternative hypothesis has the not equal operator, two tailed (left and right) test. The alternative hypothesis operator is less than which is a left tailed test. assignment essay homework paper phd report resume speech thesis