
- school Campus Bookshelves
- menu_book Bookshelves
- perm_media Learning Objects
- login Login
- how_to_reg Request Instructor Account
- hub Instructor Commons

Margin Size
- Download Page (PDF)
- Download Full Book (PDF)
- Periodic Table
- Physics Constants
- Scientific Calculator
- Reference & Cite
- Tools expand_more
- Readability
selected template will load here
This action is not available.

1.1.E: Problems in Set Theory (Exercises)
- Last updated
- Save as PDF
- Page ID 22251
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
Exercise \(\PageIndex{1}\)
Prove Theorem 1 (show that \(x\) is in the left-hand set iff it is in the right-hand set). For example, for \((\mathrm{d}),\) \[ \begin{aligned} x \in(A \cup B) \cap C & \Longleftrightarrow[x \in(A \cup B) \text { and } x \in C] \\ & \Longleftrightarrow[(x \in A \text { or } x \in B), \text { and } x \in C] \\ & \Longleftrightarrow[(x \in A, x \in C) \text { or }(x \in B, x \in C)]. \end{aligned} \]
Exercise \(\PageIndex{2}\)
Prove that (i) \(-(-A)=A\); (ii) \(A \subseteq B\) iff \(-B \subseteq-A\).
Exercise \(\PageIndex{3}\)
Prove that \[ A-B=A \cap(-B)=(-B)-(-A)=-[(-A) \cup B]. \] Also, give three expressions for \(A \cap B\) and \(A \cup B,\) in terms of complements.
Exercise \(\PageIndex{4}\)
Prove the second duality law (Theorem 2(ii)).
Exercise \(\PageIndex{5}\)
Describe geometrically the following sets on the real line: \[ \begin{array}{ll}{\text { (i) }\{x | x<0\} ;} & {\text { (ii) }\{x| | x |<1\}}; \\ {\text { (iii) }\{x| | x-a |<\varepsilon\} ;} & {\text { (iv) }\{x | a<x \leq b\}}; \\ {\text { (v) }\{x| | x |<0\}}. \end{array} \]
Exercise \(\PageIndex{6}\)
Let \((a, b)\) denote the set \[ \{\{a\},\{a, b\}\} \] (Kuratowski's definition of an ordered pair). (i) Which of the following statements are true? \[ \begin{array}{ll}{\text { (a) } a \in(a, b) ;} & {\text { (b) }\{a\} \in(a, b)}; \\ {\text { (c) }(a, a)=\{a\} ;} & {\text { (d) } b \in(a, b)}; \\ {\text { (e) }\{b\} \in(a, b) ;} & {\text { (f) }\{a, b\} \in(a, b)}. \end{array} \] (ii) Prove that \((a, b)=(u, v)\) if \(a=u\) and \(b=v\). [Hint: Consider separately the two cases \(a=b\) and \(a \neq b,\) noting that \(\{a, a\}=\) \(\{a\} .\) Also note that \(\{a\} \neq a . ]\)
Exercise \(\PageIndex{7}\)
Describe geometrically the following sets in the \(x y\)-plane. (i) \(\{(x, y) | x<y\}\); (ii) \(\left\{(x, y) | x^{2}+y^{2}<1\right\}\); (iii) \(\{(x, y)|\max (|x|,|y|)<1\}\); (iii) \(\left\{(x, y) | y>x^{2}\right\}\); (iv) \(\left\{(x, y) | y>x^{2}\right\}\); (vii) \(\{(x, y)| | x|+| y |<4\}\); (vii) \(\left\{(x, y) |(x-2)^{2}+(y+5)^{2} \leq 9\right\}\); (viii) \(\left\{(x, y) | x^{2}-2 x y+y^{2}<0\right\}\); (ix) \(\left\{(x, y) | x^{2}-2 x y+y^{2}=0\right\}\).
Exercise \(\PageIndex{8}\)
Prove that (i) \((A \cup B) \times C=(A \times C) \cup(B \times C)\); (ii) \((A \cap B) \times(C \cap D)=(A \times C) \cap(B \times D)\); (iii) \((X \times Y)-\left(X^{\prime} \times Y^{\prime}\right)=\left[\left(X \cap X^{\prime}\right) \times\left(Y-Y^{\prime}\right)\right] \cup\left[\left(X-X^{\prime}\right) \times Y\right]\); [Hint: In each case, show that an ordered pair \((x, y)\) is in the left-hand set iff it is in the right-hand set, treating \((x, y)\) as one element of the Cartesian product. \(]\)
Exercise \(\PageIndex{9}\)
Prove the distributive laws (i) \(A \cap \cup X_{i}=\bigcup\left(A \cap X_{i}\right)\); (ii) \(A \cup \cap X_{i}=\bigcap\left(A \cup X_{i}\right)\); (iii) \(\left(\cap X_{i}\right)-A=\cap\left(X_{i}-A\right)\); (iv) \((\cup X _{i} )-A=\cup\left(X_{i}-A\right)\); (v) \(\cap X_{i} \cup \cap Y_{j}=\cap_{i, j}\left(X_{i} \cup Y_{j}\right) ;\) (vi) \(\cup X_{i} \cap \cup Y_{j}=\cup_{i, j}\left(X_{i} \cap Y_{j}\right)\).

Exercise \(\PageIndex{10}\)
Prove that (i) \(\left(\cup A_{i}\right) \times B=\bigcup\left(A_{i} \times B\right)\); (ii) \(\left(\cap A_{i}\right) \times B=\cap\left(A_{i} \times B\right)\); (iii) \(\left(\cap_{i} A_{i}\right) \times\left(\cap_{j} B_{j}\right)=\bigcap_{i, j}\left(A_{i} \times B_{i}\right)\); (iv) \(\left(\cup_{i} A_{i}\right) \times\left(\bigcup_{j} B_{j}\right)=\bigcup_{i, j}\left(A_{i} \times B_{j}\right)\).
Word Problems on Sets
Word problems on sets are solved here to get the basic ideas how to use the properties of union and intersection of sets.
Solved basic word problems on sets:
1. Let A and B be two finite sets such that n(A) = 20, n(B) = 28 and n(A ∪ B) = 36, find n(A ∩ B).
Solution: Using the formula n(A ∪ B) = n(A) + n(B) - n(A ∩ B). then n(A ∩ B) = n(A) + n(B) - n(A ∪ B) = 20 + 28 - 36 = 48 - 36 = 12
2. If n(A - B) = 18, n(A ∪ B) = 70 and n(A ∩ B) = 25, then find n(B).
Solution: Using the formula n(A∪B) = n(A - B) + n(A ∩ B) + n(B - A) 70 = 18 + 25 + n(B - A) 70 = 43 + n(B - A) n(B - A) = 70 - 43 n(B - A) = 27 Now n(B) = n(A ∩ B) + n(B - A) = 25 + 27 = 52
Different types on word problems on sets:
3. In a group of 60 people, 27 like cold drinks and 42 like hot drinks and each person likes at least one of the two drinks. How many like both coffee and tea?
Solution: Let A = Set of people who like cold drinks. B = Set of people who like hot drinks. Given (A ∪ B) = 60 n(A) = 27 n(B) = 42 then;
n(A ∩ B) = n(A) + n(B) - n(A ∪ B) = 27 + 42 - 60 = 69 - 60 = 9 = 9 Therefore, 9 people like both tea and coffee.
4. There are 35 students in art class and 57 students in dance class. Find the number of students who are either in art class or in dance class.
• When two classes meet at different hours and 12 students are enrolled in both activities. • When two classes meet at the same hour. Solution: n(A) = 35, n(B) = 57, n(A ∩ B) = 12 (Let A be the set of students in art class. B be the set of students in dance class.) (i) When 2 classes meet at different hours n(A ∪ B) = n(A) + n(B) - n(A ∩ B) = 35 + 57 - 12 = 92 - 12 = 80 (ii) When two classes meet at the same hour, A∩B = ∅ n (A ∪ B) = n(A) + n(B) - n(A ∩ B) = n(A) + n(B) = 35 + 57 = 92
Further concept to solve word problems on sets:
5. In a group of 100 persons, 72 people can speak English and 43 can speak French. How many can speak English only? How many can speak French only and how many can speak both English and French?
Solution: Let A be the set of people who speak English. B be the set of people who speak French. A - B be the set of people who speak English and not French. B - A be the set of people who speak French and not English. A ∩ B be the set of people who speak both French and English. Given, n(A) = 72 n(B) = 43 n(A ∪ B) = 100 Now, n(A ∩ B) = n(A) + n(B) - n(A ∪ B) = 72 + 43 - 100 = 115 - 100 = 15 Therefore, Number of persons who speak both French and English = 15 n(A) = n(A - B) + n(A ∩ B) ⇒ n(A - B) = n(A) - n(A ∩ B) = 72 - 15 = 57 and n(B - A) = n(B) - n(A ∩ B) = 43 - 15 = 28 Therefore, Number of people speaking English only = 57 Number of people speaking French only = 28
Word problems on sets using the different properties (Union & Intersection):
6. In a competition, a school awarded medals in different categories. 36 medals in dance, 12 medals in dramatics and 18 medals in music. If these medals went to a total of 45 persons and only 4 persons got medals in all the three categories, how many received medals in exactly two of these categories?
Solution: Let A = set of persons who got medals in dance. B = set of persons who got medals in dramatics. C = set of persons who got medals in music. Given, n(A) = 36 n(B) = 12 n(C) = 18 n(A ∪ B ∪ C) = 45 n(A ∩ B ∩ C) = 4 We know that number of elements belonging to exactly two of the three sets A, B, C = n(A ∩ B) + n(B ∩ C) + n(A ∩ C) - 3n(A ∩ B ∩ C) = n(A ∩ B) + n(B ∩ C) + n(A ∩ C) - 3 × 4 ……..(i) n(A ∪ B ∪ C) = n(A) + n(B) + n(C) - n(A ∩ B) - n(B ∩ C) - n(A ∩ C) + n(A ∩ B ∩ C) Therefore, n(A ∩ B) + n(B ∩ C) + n(A ∩ C) = n(A) + n(B) + n(C) + n(A ∩ B ∩ C) - n(A ∪ B ∪ C) From (i) required number = n(A) + n(B) + n(C) + n(A ∩ B ∩ C) - n(A ∪ B ∪ C) - 12 = 36 + 12 + 18 + 4 - 45 - 12 = 70 - 57 = 13
Apply set operations to solve the word problems on sets:
7. Each student in a class of 40 plays at least one indoor game chess, carrom and scrabble. 18 play chess, 20 play scrabble and 27 play carrom. 7 play chess and scrabble, 12 play scrabble and carrom and 4 play chess, carrom and scrabble. Find the number of students who play (i) chess and carrom. (ii) chess, carrom but not scrabble.
Solution: Let A be the set of students who play chess B be the set of students who play scrabble C be the set of students who play carrom Therefore, We are given n(A ∪ B ∪ C) = 40, n(A) = 18, n(B) = 20 n(C) = 27, n(A ∩ B) = 7, n(C ∩ B) = 12 n(A ∩ B ∩ C) = 4 We have n(A ∪ B ∪ C) = n(A) + n(B) + n(C) - n(A ∩ B) - n(B ∩ C) - n(C ∩ A) + n(A ∩ B ∩ C) Therefore, 40 = 18 + 20 + 27 - 7 - 12 - n(C ∩ A) + 4 40 = 69 – 19 - n(C ∩ A) 40 = 50 - n(C ∩ A) n(C ∩ A) = 50 - 40 n(C ∩ A) = 10 Therefore, Number of students who play chess and carrom are 10. Also, number of students who play chess, carrom and not scrabble. = n(C ∩ A) - n(A ∩ B ∩ C) = 10 – 4 = 6
Therefore, we learned how to solve different types of word problems on sets without using Venn diagram.
● Set Theory
● Sets Theory
● Representation of a Set
● Types of Sets
● Finite Sets and Infinite Sets
● Power Set
● Problems on Union of Sets
● Problems on Intersection of Sets
● Difference of two Sets
● Complement of a Set
● Problems on Complement of a Set
● Problems on Operation on Sets
● Word Problems on Sets
● Venn Diagrams in Different Situations
● Relationship in Sets using Venn Diagram
● Union of Sets using Venn Diagram
● Intersection of Sets using Venn Diagram
● Disjoint of Sets using Venn Diagram
● Difference of Sets using Venn Diagram
● Examples on Venn Diagram
8th Grade Math Practice From Word Problems on Sets to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math . Use this Google Search to find what you need.
New! Comments
- Preschool Activities
- Kindergarten Math
- 1st Grade Math
- 2nd Grade Math
- 3rd Grade Math
- 4th Grade Math
- 5th Grade Math
- 6th Grade Math
- 7th Grade Math
- 8th Grade Math
- 9th Grade Math
- 10th Grade Math
- 11 & 12 Grade Math
- Concepts of Sets
- Probability
- Boolean Algebra
- Math Coloring Pages
- Multiplication Table
- Cool Maths Games
- Math Flash Cards
- Online Math Quiz
- Math Puzzles
- Binary System
- Math Dictionary
- Conversion Chart
- Homework Sheets
- Math Problem Ans
- Free Math Answers
- Printable Math Sheet
- Funny Math Answers
- Employment Test
- Math Patterns
- Link Partners
- Privacy Policy

Recent Articles
Estimating Sum and Difference | Reasonable Estimate | Procedure | Math
May 22, 24 06:21 PM
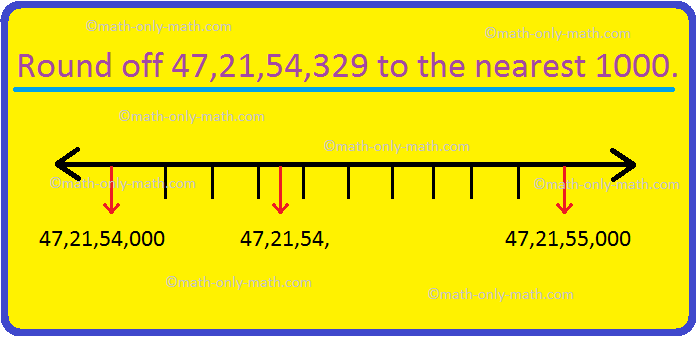
Round off to Nearest 1000 |Rounding Numbers to Nearest Thousand| Rules
May 22, 24 06:14 PM
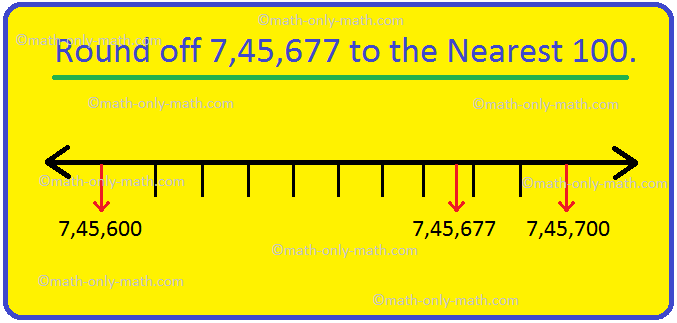
Round off to Nearest 100 | Rounding Numbers To Nearest Hundred | Rules
May 22, 24 05:17 PM
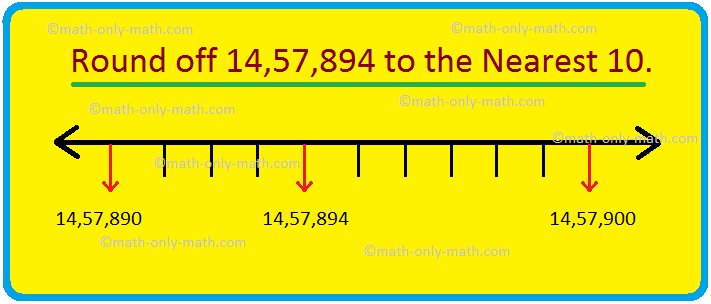
Round off to Nearest 10 |How To Round off to Nearest 10?|Rounding Rule
May 22, 24 03:49 PM
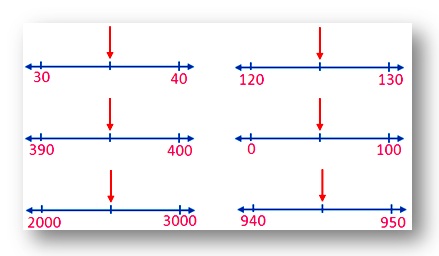
Rounding Numbers | How do you Round Numbers?|Nearest Hundred, Thousand
May 22, 24 02:33 PM
© and ™ math-only-math.com. All Rights Reserved. 2010 - 2024.
Category:Set Theory Problems
This page lists all of the problems which have been classified as set theory problems.
Subcategories
This category has only the following subcategory.
- Introductory Set Theory Problems
Something appears to not have loaded correctly.
Click to refresh .

IMAGES
VIDEO
COMMENTS
Roughly speaking, a set is a collection of objects. The objects can be anything: numbers, letters, libraries that have at least 20 male staff, or absolutely nothing. Order does not matter. What does matter is what is in the set. There might be a finite number of objects in the set, in which case it is called a finite set.
Pages in category "Set theory" The following 26 pages are in this category, out of 26 total. A. Ascending chain condition; Axiom of choice; C. Cardinality; Complement; E. Element; Empty set; F. ... Art of Problem Solving is an ACS WASC Accredited School. aops programs. AoPS Online Beast Academy AoPS Academy. About. Our Team Our History Jobs ...
A set with a single element has two subsets, the empty set and the entire set. Thus . A set with two elements has four subsets, and . Similarly, for any finite set with elements, the power set has elements. Size Comparison. Note that for any nonnegative integer, and so for any finite set , (where absolute value signs here denote the cardinality of
A set with no elements is known as the empty set (note the definite article). There are as many singletons as there are elements in your universe. They aren't the same though, for example 1 ≠ {1} 1 ≠ { 1 }. There is only one empty set and it is denoted ∅ ∅ - irrespective of the universe we are working in.
for doing mathematics, set theory provides the material for building mathematical structures. Set theory is not the only possible framework. More recently one has used category theory as a foundation. Cantorian set theory has turned out to be accessible. During the \new math" revolution the language has been introduced already for young kids.
In The Art and Craft of Mathematical Problem Solving, award-winning Professor Paul Zeitz conducts you through scores of problems at all levels of difficulty.More than a bag of math tricks, these 24 lectures reveal strategies, tactics, and tools for overcoming mathematical obstacles in fields such as algebra, geometry, combinatorics, and number theory.
Set theory is a branch of math dealing with sets which are collections of objects. However, set theory is usually applied to objects pertaining to mathematics. This article is a stub. Help us out by expanding it. Categories: Stubs. Set theory. Definition. Art of Problem Solving is an.
Art of Problem Solving offers two other multifaceted programs. Beast Academy is our comic-based online math curriculum for students ages 6-13. And AoPS Academy brings our methodology to students grades 2-12 through small, in-person classes at local campuses. Through our three programs, AoPS offers the most comprehensive honors math pathway ...
cardinality of a set, 247 Cartesian coordinates, 143 ceiling function, 193 center in coordinates, 150 centroid, 94, 137 in coordinates, 152, 153 chord, 81, 155 circle, 82 / 267. Excerpt from "Art of Problem Solving Volume 1: the Basics" ©2014 AoPS Inc. www.artofproblemsolving.com ... null set, 247 number theory, 39 numerator, 75 octagon area ...
The set \(\boldsymbol{\theta}=\{x: x \neq x\}\) is called the empty set.This set clearly has no elements. Using Theorem 1.1.1, it is easy to show that all sets with no elements are equal.Thus, we refer to the empty set.. Throughout this book, we will discuss several sets of numbers which should be familiar to the reader:
E. 10. Exercise 1.1.E. 1 1.1. E. 1. Prove Theorem 1 (show that x x is in the left-hand set iff it is in the right-hand set). For example, for (d), ( d), x ∈ (A ∪ B) ∩ C [x ∈ (A ∪ B) and x ∈ C] [(x ∈ A or x ∈ B), and x ∈ C] [(x ∈ A, x ∈ C) or (x ∈ B, x ∈ C)]. x ∈ ( A ∪ B) ∩ C [ x ∈ ( A ∪ B) and x ∈ C] [ ( x ...
In The Art and Craft of Mathematical Problem Solving, award-winning Professor Paul Zeitz conducts you through scores of problems at all levels of difficulty.More than a bag of math tricks, these 24 lectures reveal strategies, tactics, and tools for overcoming mathematical obstacles in fields such as algebra, geometry, combinatorics, and number theory.
High School Olympiads. AoPS Curriculum. The Art of Problem Solving mathematics curriculum is designed for outstanding math students in grades 5-12. Our texts offer broader, deeper, and more challenging instruction than other curricula. Our Beast Academy elementary school curriculum covers grades 1 through 5. VIEW ALL BOOKS.
Art of Problem Solving AoPS Online. Math texts, online classes, ... Category:Set theory. This is a list of articles dealing with set theory. Pages in category "Set theory" The following 26 pages are in this category, out of 26 total. A. Ascending chain condition; Axiom of choice; C.
We say a set is a subset of another set if every element of is also an element of , and we denote this by .The empty set is a subset of every set, and every set is a subset of itself. The notation emphasizes that may be equal to , while says that is any subset of other than itself. In the latter case, is called a proper subset. The following is a true statement:
Example: Determining Whether a Set Is Well Defined (cont) Solution. a) A = {x : x. is a winner of an Academy Award} This set is well defined because we can always determine whether or not a person belongs to set . A. Leonardo DiCaprio, Felicity Jones, and Ethan Hawke are members of set . A, but Hillary Clinton, Harry Potter, and Drake are not ...
The Art of Problem Solving, Volume 1, is the classic problem solving textbook used by many successful MATHCOUNTS programs, and have been an important building block for students who, like the authors, performed well enough on the American Mathematics Contest series to qualify for the Math Olympiad Summer Program which trains students for the United States International Math Olympiad team.
Apply set operations to solve the word problems on sets: 7. Each student in a class of 40 plays at least one indoor game chess, carrom and scrabble. 18 play chess, 20 play scrabble and 27 play carrom. 7 play chess and scrabble, 12 play scrabble and carrom and 4 play chess, carrom and scrabble.
Art of Problem Solving AoPS Online. Math texts, online classes, and more for students in grades 5-12. Visit AoPS Online ‚ Books ... Pages in category "Introductory Set Theory Problems" This category contains only the following page. 2. 2013 AMC 12A Problems/Problem 20;
This Art of Problem Solving Introduction to Number Theory Books Set includes 2 1. Art of Problem Solving Introduction to Number Theory Text 9781934124123 2. Art of Problem Solving Introduction to Number Theory Solutions Manual 9781934124130. Read more Report an issue with this product or seller. Previous page.
Art of Problem Solving AoPS Online. Math texts, online classes, and more for students in grades 5-12. Visit AoPS Online ‚ Books ... This page lists all of the problems which have been classified as set theory problems. Subcategories. This category has only the following subcategory. I.